
Simply stated, the challenge of a packing puzzle is to fit a given set of pieces into a container. The boundaries are either enforced by walls and a lid, or sometimes just walls, with the "lid" implied by the requirement that no piece extends beyond the level of the walls. The container might also be more of a tray, especially if the pieces don't stack in 3 dimensions. Now, if you consider this task in the abstract, the entire container could be construed as implied rather than physical, and then many assembly puzzles could be considered to be packing puzzles. For example, the SOMA cube could be re-cast as "fit the pieces into a cubic box." In addition, you can shoehorn dissections in here by thinking of the original form as the "container" - the objective is to re-construct the original form, which is tantamount to fitting the pieces back into this abstract container. For my purposes here, I will include a puzzle in the "packing" category if there is a physical container, and some pieces to cram into it. In rare instances the container is similar to the pieces themselves. Sometimes the puzzle is presented with a subset of all the pieces except for one of them packed into the container, with seemingly no room for the additional piece, and the objective being to rearrange the pieces to make the last piece fit, too. Take a look at Erich Friedman's Packing Center. Bill Cutler has written an interesting essay on box packing puzzles. In addition to his seminal designs of Interlocking puzzles, Stewart Coffin has designed many great packing puzzles. When Coffin's designs appear in the tables below, I have highlighted them like this.
 Hercules - B&P Designed by Jean Claude Constantin Nice quality and poses just the right amount of challenge. |
Crazy L A very nice little packing challenge, from the Puzzle and Craft Factory. |
 Four T's - Binary Arts/Thinkfun |

Pack the Tray (8 triangles + 1 rectangle) - Saul Bobroff I got this prototype from Saul at the 2009 NYPP. |
|||
 Houses and Factories Designed by Richard Hess - distributed by B & P |

Houses and Factories 2 - Hess Purchased at a get-together. |

Foxes and Wolves Designed by Richard Hess. Purchased at IPP 29 in SF. |
 Packing Quarters - B&P |
|||
 Butterfly - Nature's Spaces Fit nine identical penta-hexes into a triangular frame. Only one arrangement will work. |
 Frog Pond - Nature's Spaces Fit nine identical tetra-hexes into a triangular frame. |
|||||
 3 Ls Fit the 3 L-shaped pieces into the tray. |
 Lucky 7 - Melissa & Doug |
|||||
|
Blockade - B&P Blockade is like Lucky 7 - both use 3 small and 4 large L shaped pieces, but Blockade also has pins on the board and corresponding holes in the pieces. Lucky 7 is trivial to solve - Blockade adds a little (but not much) challenge. |
 Kinato Kinato is a very nicely packaged puzzle from Ravensburger. Sixteen triangles are threaded via clever swivel connections. Arrange them into a large triangle with the proper pattern. I found it at jigsawjungle.com. |
|||||

Snake Pool Eleven cubes are loosely strung along an elastic to form a cube snake. Fit the snake flat in the tray - the "pool." There are at least four different solutions. The cubes are 3/4", the tray opening is 3.25" square. The snake configuration is: 3+2+2+2+1+1 (where a + denotes a right-angled bend that can swivel). Erich Friedman shows various square in square packings on his Packing Center site, but I don't think the solution shown for 11 squares works with this particular cube snake configuration. |
||||||

Ampelmann - Roman Götter and Peter Knoppers Purchased from Roman at IPP31 in Berlin A black case with a hidden complex interior and two circular openings. Three red "Don't Walk" Ampelmann figures, and three green "Walk" figures (one mirror image or the other two), colored on only one side. Two challenges: 1) place all six figures in one "compartment" with one red Ampelmann in the middle, and 2) place all six figures in the other "compartment" with one green Ampelmann in the middle. The clear piece is a hint - it shows the shape of the cavity inside the case. Simple, eh? These figures are the old East Berlin crosswalk signal symbols - one of the few vestiges of Communist rule that Berlin citizens want to keep. Read more about " Speciation and Competition in Berlin's Traffic Lights." |
||||||



Mimi packing puzzles: A, F, H |

Pack the four T-shaped pieces into the tray - I obtained this from George and Roxanne Miller but I don't know its name. |
|||||


Modest Hexominoes by Dr. Richard Hess (IPP17) Place all 20 pieces so that each hexomino shape contains five identical pieces. Includes a booklet with 100 additional problems to maximally cover polyomino shapes with congruent tiles. |

The Massai packing puzzle from Siebenstein Spiele, 2011. Pack the 5 identical L-shaped tetrominoes in the tray. My copy might be defective, but I found one solution and my wife and kids found two more. |
|||||

Quartet in F - Stewart Coffin (#253) |
 Octet in F, designed and made by Stewart Coffin, exchanged at IPP32 by Rosemary Howbrigg |

FN Puzzle - pack the four pieces in the tray in three different ways designed by Mitsuhiro Odawara produced by Toshiyuki Kotani Purchased at IPP32 |

Retrofit - designed by Goh Pit Khiam, made by Eric Fuller, from Rosewood, Ipe, Walnut, Bubinga, Padauk, Maple, and Acrylic. |
|||

Almost There - designed by Goh Pit Khiam, issued at IPP35 in Ottawa. Fit the pieces in the tray. |
||||||
|
The following tray-packing puzzles were all designed by
Edi Nagata.
Edi sells versions in 2-sided trays, made from MDF. A couple were offered by Bits and Pieces with wooden 2-sided trays and aluminum pieces, other single-sided versions in CD cases by Embrain via Torito. |
||||||


Pencil Case |


Cat Case aka Cats in a Cradle - B&P |
|||||




Shirt Case Purchase the 2-sided MDF version from Edi, or the single-sided CD-case versions "Shikoku" and "Australia" from Torito. Philos offers a version, too. |
||||||
|
||||||
One event at the International Puzzle Party (IPP) is called the Edward Hordern Puzzle Exchange.
Qualifying attendees can sign up to participate - each must submit a new puzzle design,
and if approved, bring enough copies of the puzzle to exchange one with each other participant (up to 100).
IPP32 in Washington D.C. in 2012 was the first time I participated in the exchange.
There were 79 puzzles in the exchange in 2012.
For the exchange, I created a tray-packing puzzle
I call Non-Convex Bi-Half-Hexes. Catchy and mellifluous, eh?
I chose to use a subset of the
hexiamonds as pieces.
If one divides a regular hexagon in half along a line connecting opposite vertices,
then re-joins the two halves along a side-length, there are only seven resulting shapes that are non-convex.
Using the "standard" piece names, this set of seven includes
{ butterfly, chevron, crook, hook, snake, sphinx, yacht }.
The other five hexiamonds are either convex { rhomboid, hexagon }, or not composed of two half-hexes
{ crown, pistol, lobster }.
I used this set of seven (mathematically complete given the defining rule)
and designed four different simple symmetric trays into which all seven pieces can be packed flat (allowing gaps),
three of which have only one solution apiece.
The puzzle was produced by Steve Kelsey.
The case cover is made from a single piece of wood, with a clever laser-cut flexible "binding."
We designed a nice dovetail closure.
From what folks tell me, this is a difficult puzzle.
If you are interested in purchasing a copy, please email me at the address on my home page.
|
|||||||||||||||||

I designed an expansion set for my IPP32 exchange puzzle Non-Convex Bi-Half-Hexes. I have come up with a dozen new tray shapes into which all seven pieces will fit flat. Difficulty ranges from easy to hard, with several having only 1 or 2 solutions, but a few having 7, 9, and 12 solutions. All of the trays are "hollow" and require no internal islands. |
|||||||||||||||||
 Nine Squared - Tom Lensch All nine pieces have identical thickness but each has a different combination of length and width selected from discrete increments within a narrow range. When arranged correctly into the tray they simply drop in and out with no binding. Several incorrect packings seem like they should fit, if only you press down a little... wrong! |
|||||||||||||||||

Apothecary's Cabinet - Constantin (purchased at GPP) Each "drawer" has a combination of side tabs and portions of the row separators, and is equivalent to a rectangle with each side having either a tab or a notch. There are 2^4=16 possible arrangements including rotations and reflections. The knobs on the drawers require the reflections. The fact that the side tabs/notches are off-center requires the rotations. This puzzle is a nice realization of a 4x4 heads/tails edgematching puzzle, but includes a cabinet/tray/frame which constrains the solution, since it has all notches along the left and top, and all tabs along the right and bottom. If you assign a 4-bit binary ID to each drawer using 0 for a notch and 1 for a tab, the low bit for the top and high for the left side, then one solution is:
|
|||||||||||||||||
 Digits - Constantin Fit the 10 digits into the tray. |
|||||||||||||||||


The much-copied Digigrams, designed by Martin Watson. Made by Eric Fuller, from Grandillo, Walnut, and laser-cut acrylic. |
|||||||||||||||||

Num3er Cruncher - Mick Guy Mick is Vice President of the British Origami Society, and he kindly sent me a copy of his "Num3er Cruncher" puzzle. Thanks, Mick! Packing digits in a tray (or box) has been done before, but Mick's design is a bit different. |
|||||||||||||||||

Square Dance - designed by Derrick Schneider Purchased from Pavel Curtis - I've been wanting one for a while and was pleased to find Pavel had resuscitated it! Square Dance won an Honorable Mention in the 2002 IPP Design Competition. There is only one way to join two 2x2 squares by a half edge, and only four ways to join a third 2x2 square by a half edge to the first two. These are the four pieces of Square Dance, and there is only one way to pack them into an 8x8 tray, and only one way to pack them into a 7x9 rectangle. The included tray is two-sided. |
|||||||||||||||||
 Partridge Puzzle by Robert Wainwright obtained from Robert at the 2007 NYPP Kadon offers some of Erich Friedman's "Partridge" puzzles. In an "anti-Partridge" puzzle, there is one largest piece, and the count goes up as the pieces shrink. |

Windmill Key - Tyler Somer I received this at the 2014 Rochester Puzzle Party (RPP) that followed IPP34. Thanks, Tyler! |
||||||||||||||||

Lonpos Cosmic Creatures |
 Pentagon Tiles, designed and exchanged at IPP32 by Rene Dawir, made by Marcel Gillen |
||||||||||||||||
 13 Triangles, designed and exchanged at IPP32 by Ed Pegg Jr., made by William Waite |
 Di-Half-Hexes, designed and exchanged at IPP32 by Peter Knoppers, made by Buttonius Puzzles & Plastics I was really surprised to see this one, since it is so similar to my Non-Convex Bi-Half-Hexes IPP32 exchange puzzle. What are the odds? Peter and I must have been hit by a similar brain wave. Fortunately, his puzzle uses a different set of hexiamonds and different trays. |
||||||||||||||||


Triangle Edges - designed by William Waite in 2005 Pack the 12 pieces into the tray. The puzzle is based on a triangular grid and each piece is composed of five edges. |
|||||||||||||||||

Square Dissection - N. Baxter Received from Dr. R. Hess at a get-together - thanks, Dick! |

Domino Peg From PuzzleMist (William Waite) Fit the 12 pieces in the tray to form different patterns of holes - ten goal patterns specified on the back of the tray. |
||||||||||||||||

Bugs - designed by Alexander Magyarics and made by Pelikan from Pink Oak and other hardwoods. Fit the four bugs flat in the obstructed frame. Has a nice acrylic cover for display. |
|||||||||||||||||
 Karin's Star Cluster An entry in the IPP24 Design Comp.. |
 Tessellating Galaxies - JVK |
 Sun Dance - JVK |
 Fantastic Island |
|||||||||
 The City 2001 Binary Arts (Thinkfun) Pack six heptominoes (3 distinct pieces and their mirror images) in the 6x7 tray. Nice metal pieces with 3D abstract buildings on them which prevent the pieces from being flipped and exclude most of the otherwise possible 80 assemblies. |
||||||||||||
 Fit To A Tee - Thinkfun A nice metal tray-packing puzzle from Thinkfun. Pack the 9 pieces representing golf holes complete with tees, sand traps, and pins, into the base. The base presents a challenge on each side (the front and back nines), with different arrangements of fixed water hazards to work around. Oh, and just as on a real course, abut each flag with the tee of the next hole! |
||||||||||||
 Geometrex Set - Ormazd, Nabucho, and Quirinus In each case the pieces can be rearranged within the tray to fit in an extra square. |
 The "845 Combinations" puzzle is almost like pentominos... here is a solution to the 845 puzzle. |
|||||||||||


On 6/22-25/13, made a trip to Niagara Falls. At Niagara Falls, I stopped in at Turtle Pond Toys. They carry several nice puzzles of various types. I picked up the IQ Puzzle from Toyland Company. It has 9 curved pieces that must be fit into the channels within a 4x4 grid of circles. It is an easier version of the 845 Combinations puzzle, which has 10 pieces to be fit into the same grid. |
||||||||||||
 Adam's Cube |
 One Way |
 Boxed In - Milton Bradley |

The IQ Link puzzle from Smart Games designed by Raf Peeters |
|||||||||
 Circle Challenge - Melissa & Doug A good one for kids - work on it from the inside out. The pictures on the pieces are merely decorative. |
 Double Cross - Mag Nif There are four pink plastic pieces and the tray. The objective is to form a cross (plus sign) in the tray. |
|||||||||||
 Magic Block (MCS promo) |
 Figa Block |
 IQ Block |

Quartet Quandary From PuzzleMist (William Waite) Fit the four pieces in the tray. Two solutions. |
|||||||||

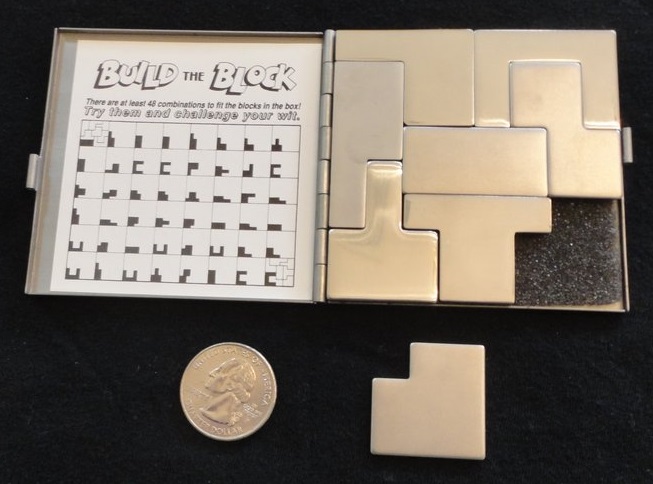
Build the Block - a metal square dissection puzzle branded "Arco." |
||||||||||||
 Sleazier - Pavel Curtis based on Stewart Coffin's Four Sleazy Pieces (#169A) Fit the 4 pieces into the tray. IPP25 |
 Stewart Coffin's Sunrise / Sunset (#181) Fit the 5 polyominoes into each side of the tray, making a symmetric pattern in each case. Gift from Bernhard Schweitzer (thanks!). IPP22 |
 Stewart Coffin's Drop In (#153B) aka The Trap Fit the four pieces into the box through a small slot. They must be arranged so all fit within the inside perimeter of the box walls. Saul Bobroff IPP23 |
 Stewart Coffin's Few Tile (#133) Made by John Devost A beautiful Padauk frame about 5.75" squared, with corner splines, and Birch plywood pieces. A gift - Thanks, John! |
|||||||||
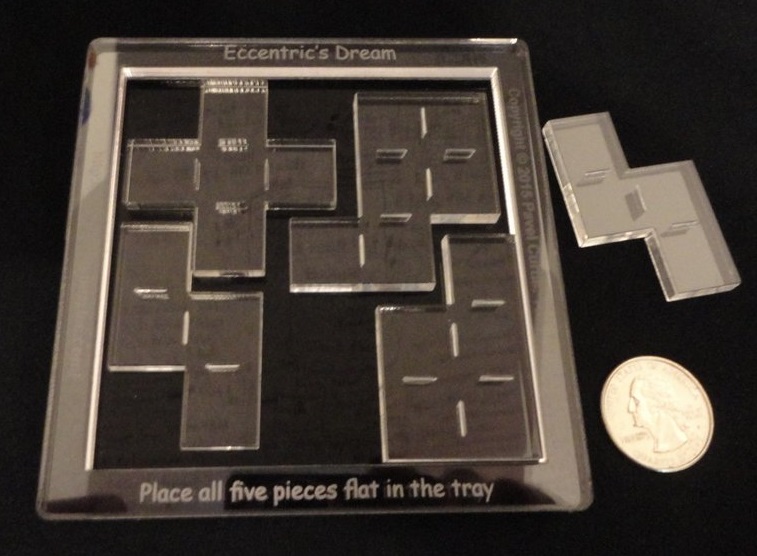
Eccentric's Dream - designed and made by Pavel Curtis |

Stewart Coffin House Party (#250) Fit the four Marblewood pieces into each side of the tray, which is made from Poplar on Baltic Birch. Made by Tom Lensch |
|||||||||||
 Stewart Coffin's Four Fit (#217) Made by Tom Lensch. Purchased from Tom at the Dartmouth College Mechanical Puzzle Day in Feb. 2008. |

Stewart Coffin's Five Fit From Dave Janelle at Creative Crafthouse. Fit the five pentomino pieces into the square tray. The tray has a handy storage space for one of the pieces should you be unable to solve it. |
|||||||||||

A Stewart Coffin Tray Puzzle Set (#181), in Poplar and Lyptus woods, made by Tom Lensch. Purchased at Puzzle Paradise .ca. This set includes six of Coffin's tray-packing puzzles - a single-sided rectangular tray (#181, 1 solution), a two-sided pentagonal tray (#181-C, The Housing Project, 1 solution each side), and another two-sided pentagonal tray having a movable wall segment on one side (#181-A, The Castle Puzzle, 3 solutions; #181-B, The Tree Puzzle, 2 solutions, other side #181-B, The Vanishing Trunk Puzzle, 1 solution). |
||||||||||||
 Stewart Coffin's Cruiser (#167) Made by Walter Hoppe. |

Lean 2, designed by Stewart Coffin, made by Tom Lensch, exchanged at IPP32 by Dave Rossetti |

Buridan, designed, made, and exchanged at IPP32 by Vladimir Krasnoukhov |

Heart and Bud, designed, made, and exchanged at IPP32 by Yoshiyuki Kotani |
|||||||||
|
||||||||||||
 Think Square - Pressman There are 4 small right triangles, 4 large right triangles, 4 stair-case shaped pieces, and 5 small squares. The pieces can be fit snugly into the tray with and without one of the five small squares. |
 Triaden spass - Logika |
 Pack It In - Great American Puzzle Factory 1996 Pack a set of 16 items into a suitcase frame. Flat cardboard pieces. |
 The Trapped Man - Tom Jolly Laser cut by Walter Hoppe. Five unusually convoluted pieces, including the little "man." The first challenge is to fit them into the tray so that none can slide or rotate. Next, try it with only four of the five pieces, then with only three! Several other puzzle goals accompany the Trapped Man puzzle. |
|||||||||



The Suitcase Puzzle - made in China for Bear, Bear, & Bear Ltd. England 1996 |
||||||||||||
 Pac-Man - Milton Bradley First create 4 Pac-men with open mouths. Then use the same pieces to create 3 Pac-men with closed mouths. There are eye stickers on some pieces, which must be positioned correctly. The pieces can be flipped. |
 The Jayne Fishing Puzzle - A 1916 advertisement of Jayne's Tonic Vermifuge (yuck!). Discussed in Slocum and Botermans' "The Book of Ingenious and Diabolical Puzzles" on page 15. You were to cut out the fish and the ring and then pack the fish inside the ring. The fish names are (left to right, top down): Codfish, Shad, Red Grouper, Cowtrunk Fish, Flying Fish, Bluefish, Mackerel, Tarpon, Sheepshead, Moonfish, Striped Bass, and Weakfish. Also see No Fishing by Bepuzzled. |
 No Fishing - Bepuzzled 1998 Remove the water then fit all twelve fish into the bowl. This is a nice wooden laser-cut, colorful, and faithful copy of the Jayne Fishing Puzzle of 1916. |
||||||||||
 In the Raging Rapids puzzle from Thinkfun (Binary Arts), you have to fit all the men into the raft, facing the right way. The figures' bases have various patterns of tabs and indents. |
 In the Mayan Calendar puzzle from William Waite, you have to fit all the glyphs into the tray, facing the right way. The glyphs have various patterns of tabs and indents. (Similar to Raging Rapids.) |
Alex Randolph's Moebies - Springbok 1973 There are 8 sockets at various positions in the orange board. Six pieces and six pegs are included - the object is to find a way to peg the six pieces to the board so that all fit within the edges. |
 Springbok Fitting & Proper |
|||||||||
Here is a nice set of small, pocketsized tray packings designed by William Waite, purchased from his
PuzzleMist website:




 From left to right, they are: Triangle Quorn, Square Quorn, Hex Quorn, Diamond Teaser, and Mix Teaser 2.
From left to right, they are: Triangle Quorn, Square Quorn, Hex Quorn, Diamond Teaser, and Mix Teaser 2.
|
||||||||||||


The Kitchen Ceiling Puzzle - designed by William Waite in 2006 Pack the 12 pieces into the tray so that the holes make symmetric patterns. |


Optimal Tumble - designed by William Waite in 2010 Pack the 12 pieces into the tray so that the holes make symmetric shapes. |
|||||||||||





Vintage 1969 packing puzzles from Lakeside. So far they include:
|
||||||||||||

 JVK Tessellating Hexagons |
 Galaxies & Stars - JVK |
 "Tripple 7" - 3-piece packing (prototype) - JvK |
||||||||||

Easy Eight / Hard Eight - Bob Hearn |
 Wetten Dass... Also known as FACT Purchased in Berlin. The tray has a moving bar, pivoted at one corner. When the bar is aligned along the top edge, the five pieces are easy to pack into the tray. When the bar is aligned along the side edge, it's more difficult. |

Toysmith 11 pc. wood puzzle |
 Mind the Gap - Chris Morgan |
|||||||||
Some tray packing puzzles designed by Naoyuki Iwase (Osho) -
Mouse, Tulip, and Seals:


 See Osho's website,
Puzzle-In.
See Osho's website,
Puzzle-In.
|
||||||||||||

eLeL4 - designed by Hiroshi Yamamoto presented at IPP30 by Hiroshi Uchinaka Fit the four pieces into the 8x8 tray. Each piece is composed of 2 'L' shapes. |

Unique U - designed by Hiroshi Yamamoto Fit the six U-shaped pieces into the 9x9 tray. |

The Nifty Fifty from Jean Claude Constantin requires you to pack the four pieces into the tray. |

The Quartet Puzzle - the Quartet's tray has a movable end wall, and you must pack specific subsets of pieces into the tray depending on where the wall is positioned. |
|||||||||


Four in a Frame - a two-sided four-piece tray packing puzzle based on a triangular grid, designed by Markus Götz |
||||||||||||

Pack-Man - Chris Enright |

Hexagon 10 |

Game Ball Puzzle |
||||||||||

Animals of Australia Dump out the ten nicely cut animal pieces and try to fit them back in the tray. No peeking at the solution! |

Forever Wild - Animals of the Adirondacks Pack the ten nicely laser-cut animals into the tray. The animals all go in with a specific side upwards. From Creative Crafthouse. |
|||||||||||

Forever Wild - Animals of the Appalachians - a tray packing puzzle, one of a series - each comprising a high-quality tray and nice laser-cut pieces in various colors. |

The Cook's Cupboard Puzzle Pack the eleven kitchen items into the tray. From Creative Crafthouse. |
|||||||||||



Hexus, a packing puzzle from Brainwright. Seven pieces and a movable "challenge block" to be placed on a hexagonal grid according to a series of 44 challenges. Purchased at Necker's. |
||||||||||||

Quadrillion - designed by Raf Peeters, produced by SmartGames Thanks, Raf! Arrange the four base plates per a challenge, then pack the pieces on. |

Artefacts - designed by Frederic Boucher made by Eric Fuller, from Walnut, Baltic Birch, Chechen, Maple, and Steel. The five wooden pieces pack flat in the tray with the steel dowel three ways, but with the dowel in the hole the pieces pack only one way. |
|||||||||||

The Hive Pack four honeycomb pieces in the tray, and capture the bees. Many ways to capture 3, only one way to capture all four. From Puzzleguy (Dan Diehl) |
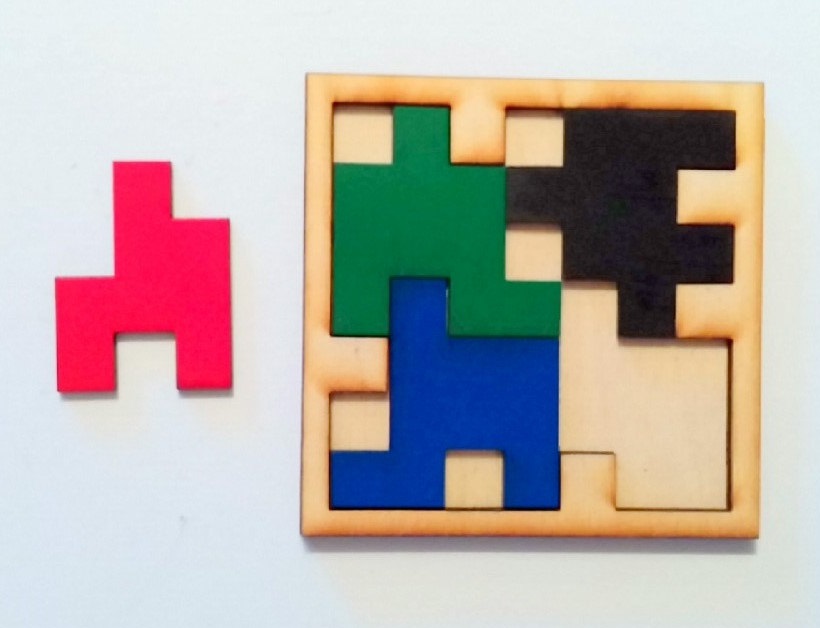
Aggravater Four piece tray packing. From Puzzleguy (Dan Diehl) |
|||||||||||

Jurassic Pack (V2) - by Jerry Loo |
I purchased four new acrylic laser-cut tray-packing puzzles from Jerry Loo -
clockwise from top left: Tick-Pack-Toe, Fish Tank (designed by Pit Khiam Goh), Fly in My Pumpkin Soup, Hash Pack
|
|||||||||||

Thinking Crow - designed by Osanori Yamamoto purchased from Jerry Loo's online store. Fit the four pieces in the tray. |

Outback - designed by Stewart Coffin, produced by Creative Crafthouse Fit the pieces flat in the tray. |
|||||||||||

Penguins Pool Party - SmartGames Tray packing on a hexagonal grid - graduated challenges using four "ice floe" pieces and up to four single-hex penguins. Charming! |

IQ XOXO - SmartGames 120 graduated challenges. Pack the 10 2-sided pentomino pieces in the tray. Where an 'O' appears on one side of a piece, an 'X' is on the opposite side, and vice versa. Bumps in the tray demand 'O' sections over them; every 'O' must be adjacent to 'X' segments, and vice versa. |
|||||||||||

Parking Puzzler - SmartGames A tray-packing puzzle with graduated challenges. |
||||||||||||

Puzzometry Jr. - Jim Fox at Puzzometry |

The 12th Tile - designed by Masaki Watanabe |
|||||||||||


Wrench Packing Puzzle - A Steam-Punk packing puzzle made by hand from used industrial tools, from the Etsy shop of Václav Skopek, Shokcz. |
||||||||||||
|
The talented Yuu Asaka of Japan has created several wonderful tray packing puzzles, each of which confounds one's preconceptions.
Some may be available from my friends at
PuzzleMaster.ca.
|
||||||||||||

Ugly4 - a perplexing jigsaw puzzle from Jigsawholic |
||||||||||||

San Diego Zoo Pandas - a packing puzzle from Brian Young, exchanged at IPP38 by Rosemary Howbrigg |

Popinjay - designed by Haym Hirsh Pack the J-shaped pieces into the tray. Exchanged by Haym at IPP39. A kind gift - Thanks, Haym! |
|||||||||||


Paulo's Enigma and Here Comes the Sun by Alexander Beresford See Alex's site Dual Brain Games, and Creative Crafthouse. Thanks, Alex! |
||||||||||||

Stable Boy - designed by George Sicherman, made by Brian Menold An anti-slide puzzle - arrange the five pieces in the tray such that none can move. (I found a "solution" using only four of the pieces.) Purchased from Brian at the New York Puzzle Party (NYPP 2020) in February. George and Brian both attended and met in person there for the first time. |
||||||||||||
|
Casey and Sean at
Pocket Pulp
[Etsy shop]
kindly sent me a selection of their puzzles, including
Pig Pen.
Thanks, Casey!
I really like Pig Pen - only six pieces but a clever challenge! |
||||||||||||

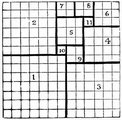 The problem of Mrs. Perkins' Quilt (or Mrs. Perkins's Quilt) appeared as no. 173 in
Henry Ernest Dudeney's 1917 book Amusements in Mathematics.
You can find the book and the problem online in a few places, including
at
www.gutenberg.org, and at
www.scribd.com.
The problem: given a large square quilt made of 13x13 small squares (169 small squares total),
find the smallest possible number of square portions of which the quilt could be composed -
i.e. a dissection of the large square into a number of smaller squares that don't all have to be different.
However, only prime dissections are allowed -
the side lengths of the component squares cannot all have a common factor - they must be relatively prime.
There can be no sub-square which is itself divided - such a solution is termed "primitive" -
primitive quilts are quilts without sub-quilts.
Martin Gardner devotes chapter 11 in his 1977 book Mathematical Carnival
to Mrs Perkins' Quilt and Other Square-Packing Problems.
Ed Pegg discussed the problem on his
Math Games site.
The problem is also discussed at
mathworld.wolfram.com.
The solution comprises 11 squares and is shown at
gutenberg.org.
It contains the following number of squares of given sizes:
1x72,
2x62,
1x42,
2x32,
3x22, and
2x12.
The smallest numbers of squares needed to create relatively prime dissections of
an n×n quilt for n=1, 2, ... are 1, 4, 6, 7, 8, 9, 9, 10, 10, 11, 11, 11, 11, 12, ...
(Sloane's A005670).
Karl Scherer discusses additional variations at
his website.
Karl defines a nowhere neat tiling - in which no two tiles have a full side in common,
and a no touch tiling - where tiles of same size cannot touch,
noting that no-touch are always nowhere-neat.
The problem of Mrs. Perkins' Quilt (or Mrs. Perkins's Quilt) appeared as no. 173 in
Henry Ernest Dudeney's 1917 book Amusements in Mathematics.
You can find the book and the problem online in a few places, including
at
www.gutenberg.org, and at
www.scribd.com.
The problem: given a large square quilt made of 13x13 small squares (169 small squares total),
find the smallest possible number of square portions of which the quilt could be composed -
i.e. a dissection of the large square into a number of smaller squares that don't all have to be different.
However, only prime dissections are allowed -
the side lengths of the component squares cannot all have a common factor - they must be relatively prime.
There can be no sub-square which is itself divided - such a solution is termed "primitive" -
primitive quilts are quilts without sub-quilts.
Martin Gardner devotes chapter 11 in his 1977 book Mathematical Carnival
to Mrs Perkins' Quilt and Other Square-Packing Problems.
Ed Pegg discussed the problem on his
Math Games site.
The problem is also discussed at
mathworld.wolfram.com.
The solution comprises 11 squares and is shown at
gutenberg.org.
It contains the following number of squares of given sizes:
1x72,
2x62,
1x42,
2x32,
3x22, and
2x12.
The smallest numbers of squares needed to create relatively prime dissections of
an n×n quilt for n=1, 2, ... are 1, 4, 6, 7, 8, 9, 9, 10, 10, 11, 11, 11, 11, 12, ...
(Sloane's A005670).
Karl Scherer discusses additional variations at
his website.
Karl defines a nowhere neat tiling - in which no two tiles have a full side in common,
and a no touch tiling - where tiles of same size cannot touch,
noting that no-touch are always nowhere-neat.
|
|
The problem of Mrs. Perkins' Quilt leads to other questions.
In general, how might it be possible to dissect various rectangles or squares into smaller squares?
Such puzzles are known as Squared Rectangles and Squared Squares.
If a dissection results in pieces all of different sizes, the dissection is called perfect, otherwise it
is imperfect.
If the dissection does not contain any smaller square or rectangle that is itself further divided, it is
called simple (or primitive), otherwise it is compound.
The order is the number of tiles used.
When describing solutions, it is convenient to use a notation called Bouwkamp code.
One lists the side lengths of the tiles as they appear in the solution, in left to right order, top to bottom,
bracketing groups with flush tops.
There is a nice article in Martin Gardner's 1962 book More Mathematical Puzzles and Diversions, in
chapter 17: Squaring the Square - by William T. Tutte,
from Gardner's November 1958 column in Scientific American.
Stuart Anderson of New South Wales has a great website called
www.squaring.net
where he discusses this topic in depth, and gives lots of historical information.
Some of the diagrams below are adapted from Stuart's site.
The topic is also discussed at
mathworld.wolfram.com.
In 1925, Zbigniew Moroń (1904-1971), of Wraclow, Poland, published a paper, 'O Rozkladach Prostokatow Na Kwadraty' (On the Dissection of a Rectangle into Squares), in which he showed a simple perfect squared rectangle (SPSR) of order 9. Reichert and Toepkin (1940) proved that a rectangle cannot be dissected into fewer than nine different squares (see Steinhaus 1999, p. 297). I have the plastic Perfect Squares (Le Carre Parfait) puzzle by Dollarama (China). It's got 9 pieces to be packed into a tray. I measured the tray cavity and the piece dimensions, and allowing for measuring error, manufacturing tolerances, and gaps so the pieces can be easily manipulated, this is an example of the Moroń 1925 SPSR.
|
Robert Wainwright presented the Partridge Puzzle at the second Gathering for Gardner, in 1996.
Partridge puzzles call for the dissection of a large square into a set of smaller squares, without voids,
such that one small square tile of size 12 is used, two of size 22 are used,
three of size 32 are used, up to n of size n2.
Kind of like the "Partridge in a Pear Tree" song, the number of square tiles of each size
increases by one at each step.
They're based on the following mathematical equivalence:

Erich Friedman also discusses Anti-Partridge tilings. In an Anti-Partridge Puzzle, one must dissect a square using n copies of a 1x1 square, (n-1) copies of a 2x2, (n-2) copies of a 3x3, etc., through 1 copy of an nxn. They're based on the mathematical equivalence: 
|
Another type of square-packing problem,
discussed by
Ed Pegg Jr.,
is to find the minimal side m of square m2
into which one can pack one of each square of sides 1, 2, 3, ..., n.
In this problem, there can be voids.
In fact, in this type of problem packing the large square without gaps is not possible.
The
only series of squares which sum to a square is for squares of sides 1 through 24,
which sum to 702 = 4900.
(This is also the only number that is both square and pyramidal - i.e. 4900 balls can make a square,
and also be stacked in a square-based pyramid with layers of 1,4,9,16, etc. -
proved by G. N. Watson in 1918.)
A proof that no perfect tiling of the 702 with squares 1-24 exists was done in 1974 using
exhaustive computer search by Edward M. Reingold (Gardner 1977).
The Sloane sequence
A005842
gives
a(n) = minimal integer m such that the m2 square contains all squares of sides 1, ..., n.
This problem has
practical applications, such as electronic circuit layout.
Minami Kawasaki gives a
catalogue of known solutions.
From Ed Pegg, here is a packing of 1-51 into a 214x214:
|

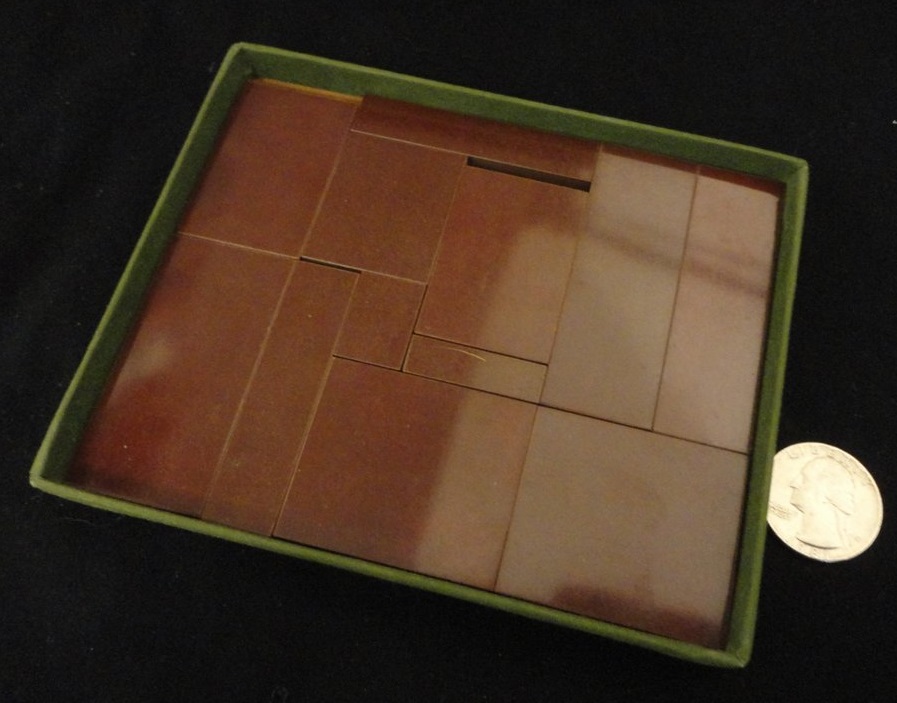


I obtained an original instance of the Calibron Twelve Block Puzzle, produced by Theodore Edison, son of the famous Thomas Edison. All twelve puzzle pieces are present and intact, but the spacer piece is missing. It was made by Calibron Products of West Orange, N.J. ca. 1932. I've been intrigued by this puzzle for some time and I thought I'd cover it here.
George Miller and Nick Baxter wrote an article The Mystery of the Calibron Twelve Block Puzzle published in the 100th issue of the CFF newsletter, in which they explore the confusion surrounding the piece dimensions. They say reportedly less than 200 units of this puzzle were sold, so it is fairly rare. One set of dimensions of the pieces are shown on Iwase's site.
If you search Google Books for calibron puzzle, you will find links to an ad for the puzzle, selling for $1, in the Jan 1935 issue of Popular Science magazine, an entry for the puzzle in the Catalog of Copyright Entries showing the puzzle was copyrighted on Dec. 22 1932, and an ad in the 1933 New Yorker Vol. 9, claiming that the puzzle has "Baffled over 900 scientists at a recent convention."
About.com says that the company Calibron Products was "established by Theodore Edison (1898-1992) [Wikipedia] [bio at nps.gov] to keep some of his late father's employees and engineers working together on research projects." Theodore's obituary in the New York Times on Nov. 26 1992, says he was the last surviving child of the inventor Thomas Alva Edison. From the inside of the box: "The problem is to arrange the twelve blocks to form a single large rectangle. Any rectangle will do, provided that all twelve blocks are used... We guarantee that there is a straightforward, accurate solution of this puzzle in a single plane, and without recourse to any kind of trick... However, in spite of the enormous number of possibilities, there appears to be only one basic arrangement which satisfies the above conditions... We once offered $25 for the first solution of this problem and distributed hundreds of puzzles at that time, - but recieved almost no correct arrangements! We should like to hear from you if you succeed in making the rectangle unaided." Here is a list of the 12 pieces, using Iwase's dimensions halved:
|
|


Yukiyasu Sekoguchi has designed many puzzles he calls "Happiness Cubes."
His designs include a
3-D version of Duijvestijn's order-21 dissection.

|
In 1978 at a conference at Miami University, Dean Hoffman posed the following problem,
which has come to be known as
Dean Hoffman's Packing Problem, or the Sugar Lump Puzzle:
Pack 27 cuboids with sides A,B,C into a box of side A+B+C, such that:
(1) A,B,C are all not equal, and
(2) the smallest of A,B,C must be larger than (A+B+C)/4.
There may be voids, but all sides will be flush.
Example dimensions are:
18,20,22 with box 603;
or 4,5,6 with box 153 (Cutler).
Cutler says there are 21 solutions, none having symmetries.
See Bill Cutler's article
Block-Packing Jambalaya.
Several examples have been produced:
by
John Devost,
by Trevor Wood,
a cheap monkeypod wood version available at
www.gemanigames.co.uk,
and a version by Trench Puzzles called The Troublesome Twenty-Seven with mahogany pieces and a flimsy plastic "box."
I acquired the latter in an auction from the Ergatoudis collection.

|


Hoffman JR - designed by Haym Hirsh, made by Tom Lensch Haym started with the 27-piece Hoffman's Packing Problem a.k.a Sugar Lump Puzzle, and combined the individual blocks from one of the 21 solutions into six composite pieces that form a cube obeying the same planar face with interstices format. Tom Lensch crafted the puzzle from 27 blocks of different exotic woods, each engraved with the wood's name, and included a nice box with a lid. Tom offered me one of a very limited edition at RPP 2019 and I snapped it up! |
|
|
Pack It In - Thinkfun This is "Conway's Curious Cube" which calls for three 1x1x1 cubes and six 1x2x2 blocks to be packed into a 3x3x3 box. There is only one solution - see this source. |

 Nine rhombic pieces fit in the tray. This is isomorphic to Conway's Curious Cube. |
 17 piece packing cube Another John Conway design. 5 of 1x1x1, 6 of 3x2x2, 6 of 1x2x4. Fit into 5x5x5. The same pattern should show on all sides. Gemani calls this "Made to Measure." I've also seen it as "Shipper's Dilemma." |
|
 Conway Box Deluxe This is a nicer version of the 17-piece Conway cube. |
 36 piece Packing Puzzle |
 T Party - B&P |
 Loyd's Cube - Sam Loyd An IPP Puzzle from Jerry Slocum |
 The Meiji Caramel puzzle is a version of Anti-Slide designed by William Strijbos. Pack 15,14,13, or 12 of the 15 1x2x2 pieces into the 4x4x4 box such that none can slide in any direction. There are no solutions using less than 12 pieces. Using 12 pieces there are only three solutions, but using 13 pieces there is only one solution. This puzzle won 2nd place in the 1994 Hikimi Wooden Puzzle Competition. Purchased from Torito. |
|||

 L-Bert Hall Pack the nine identical pieces into a 3x3x3 cube seated in the box. Each piece is a concave tri-cube with holes and one dowel. This was designed by Ronald Kint-Bruynseels for IPP27, and made by Eric Fuller. The pieces are made from Cocobolo and the box is made from Lacewood. |
|||


"The Five Minute Puzzle That Might Take a Little Longer" Designed by Andy Turner Entered in the IPP 2009 Design Competition Made by Eric Fuller, from Oak (box) and Paduak |
 Wim Zwaan - Octahedron and Tetrahedron Fit the Wenge tetrahedron into the Baltic Birch plywood Octahedral box. Then get it out again. Since the opening and the tetrahedron are not quite regular, this is more difficult than it might at first seem. Purchased from Wim at IPP28 in Prague. |
 Cubes in Space, designed and exchanged at IPP32 by Hirokazu Iwasawa (Iwahiro), made by DYLAN-Kobo An anti-slide challenge. |
|
 Mine's Cube of Cubes Designed by Mineyuki Uyematsu in 2004. Exchanged at IPP24. 14 pieces pack into a 5x5x5 box. 2 solutions. |
 Mmmm Pack the four M-shaped pieces into the box and close the lid. Designed by Hirokazu Iwasawa (Iwahiro). |
 Mmm Pack the three M-shaped pieces into the box and close the lid. Designed by Hirokazu Iwasawa (Iwahiro). |

Logs in Box designed by Vesa Timonen Produced by Hanayama in their "Woody Style" line. |
These puzzles are all based on the same design:




Aha Rectangle - Thinkfun; Log Stacker - Elverson; Logs in a Box - B&P; Lox in Box designed by Vesa Timonen 8 pieces, each with a beveled end, to be fit into the tray. |
|||
 Cherry Cocktail Pack six pieces - 3 each of 2 kinds - plus the "cherries" into the "glass." Purchased from Irina Novichkova at IPP28 in Prague. |
 Thick 'n' Thin No. 7 Purchased from Serhiy Grabarchuk at IPP28 in Prague. |


"Old Hand Cranes 1 Gin" Eight different blocks to be packed in the wooden Sake cup designed by Nob Yoshigahara produced by Hikimi |
|


Bermuda Hexagon designed by Bill Cutler in 1992 (using a computer), made by Tom Lensch 12 pieces to be packed into the hexagonal case in 3 layers. This design was awarded the 3rd prize in the 1992 Hikimi Wooden Puzzle Competition |
|||

 This is one of Trevor Wood's Teaser Tiles puzzles.
Nine tiles, each composed of two slightly different sized layers, with various overhangs.
The objective is to fit the pieces flat into the box -
i.e. so that all pieces have their two layers parallel to the
bottom of the box.
Obviously, the particular juxtaposition of piece edges and overhangs will be crucial.
Thanks, James!
This is one of Trevor Wood's Teaser Tiles puzzles.
Nine tiles, each composed of two slightly different sized layers, with various overhangs.
The objective is to fit the pieces flat into the box -
i.e. so that all pieces have their two layers parallel to the
bottom of the box.
Obviously, the particular juxtaposition of piece edges and overhangs will be crucial.
Thanks, James!
|
|||

Hoffman's Packing Box, or The Inequality of the Means Puzzle - produced by Creative Crafthouse Fit the 27 identical blocks into the frame. Based on the fact that the arithmetic mean of three values a, b, and c is always greater than or equal to the geometric mean of those values. Symbolically, (a+b+c)/3 >= (abc)^1/3 Starting with the above inequality, cube both sides - we get (a+b+c)^3 / 27 >= abc, then multiply through by 27 to get (a+b+c)^3 >= 27 * abc This means if we have 27 blocks whose dimensions are axbxc, we should be able to fit them into a cube whose edge length is (a+b+c). |

Chain Store - designed by Goh Pit Khiam Made by Tom Lensch John Rausch brought this to Brett's house for NYPP 2016 - I loved the way he introduced it as a "one piece packing puzzle." Fit the links inside the box below the rim. |
||


Spherical Packing Puzzle - designed by Laszlo Molnar, made by Eric Fuller from Walnut and Birdseye Maple |
|||


8 Blocks in a Tray - from the Etsy shop of Bill Sheckels |
|||


Nob's Never Ending Build a cube within the box, from 8 similar angled pieces. The one on the left is a rough handmade version - an auction win. I recognized this in a pic of Matti Linkola's exhibition, and found it on Trevor Wood's site. It is a copy of Nob's Neverending puzzle. Torito sells a version made by Himiki. |
 Make Room - variation of Stewart Coffin's #127, by Mr. Puzzle Australia Craftsman version in fine exotic woods - the box is a waxy wood called Yellow Leichardt. Four challenges:
|
 This is Tube-It-In by William Strijbos. (Photo from John Rausch's site.) |
 The Morph A cube dissected into four clever pieces can morph into three different solids to fill the compartments in the case. According to Bernhard Schweitzer, who sells a copy, this was designed by Boris A. Kordemsky of Russia. I believe this was issued by Bits and Pieces quite some time ago, but I am not sure. I found my copy on auction. |

Funny Cubes - designed and made by Tom Lensch. Purchased at IPP 29 in SF. Each piece consists of two attached rectangular blocks that can be rotated relative to each other. Fit the blocks into the square tray so that the top pieces also form a square. |

Devil's Gate - designed by Ferdinand Lammertink, made by George Miller Purchased from George during a visit to the Puzzle Palace during IPP 29. This is a version of the Langford problem. Find lots of info on the Langford problem here. |


Perilous Pipes designed by Ferdinand Lammertink Computer Analysis by George Miller Issued by Popular Playthings 2011
This is a commercialized graduated-challenge style version of
|
||


George Bell's Nine Bed Nightmare assembly puzzle (May be available from Puzzlewood.de.) Pieces bot. to top, L to R: A, B, C, D, E, F, G, H, I. Challenges:
|
||




Eric Harshbarger's Digits in a Box Ten size 1x3x5 digits - just pack them into a 5x5x5 box. The first version, with pieces laser-cut from colored acrylic, was exchanged at G4G9 - I purchased a copy at Eureka. Popular Playthings now offers a nice mass-produced version. |
||
 16 Hexominoes in a Twin Square, designed and made by Marcel Gillen, exchanged at IPP32 by Carlo Gitt |
 L-omino Cube 4, designed and exchanged at IPP32 by George Bell, made by Ponoko |
 O'Beirne's Cube, or "Morph 2" - designed by T.H. O'Beirne, made by New Pelikan Workshop, exchanged at IPP32 by Peter Hajek |
|
Paul Stevens of Wisconsin kindly sent me a copy of a puzzle
he designed and made called the 8-in-1 Puzzle. It is made from recycled wood and comprises eight pentacubes and a two-compartment box. All but one of the pentacubes are non-planar. Select any seven of the eight pieces then pack them into the 3x3x4 compartment, allowing a single 1x1x1 void. It is quite challenging! Thanks, Paul! 
|

Outfox the Box (245A) - designed and made by Stewart Coffin exchanged at IPP35 by Abel Garcia Fit the four pieces in the box with the lid closed. |
|

Dictionary Case - designed by Koichi Miura, issued by Chronos Co., Ltd., purchased at tocoo.com Fit the five pieces into the box. I think I solved it... |

6T - designed and 3D-printed by Ken Irvine |
|

6 More T - by George Bell at PolyPuzzles |
||
 Bunchgrass Packing Puzzle "13/14" A box with 5 pieces made of spheres - the pieces fit in the box with or without a single sphere piece. They also can form a square-based pyramid. It is called the 13-14 puzzle since with the single sphere there are 14 pieces and 13 without. |
 For Your Own Sake - Hikimi (Japan) This puzzle poses the additional challenge of embedding 3 marbles. |
 Dragon's Eggs - Pentangle Find a way to pack everything into the box so that the three "eggs" are all concealed. |
 Slot Machine - Stewart Coffin #185 obtained from Henry Strout Build a cube within the box, fitting the pieces in through a small slot in the acrylic cover. |
 18-Piece Mini-Cube-Block Puzzle Set |
 Four Square Fit the four dual-layer pieces into the tray. |

Back in the Box A dissection of a cube into various tetrahedra. |
 Mosaic - IQ Puzzles (Family Games) |
 Third Degree - Bits and Pieces Designed in 1995 by Bill Cutler, who calls it the "3-Piece Blockhead." Discontinued. |


Stark Raving Cubes / Sneaky Squares / Blockhead / Blockout I bought mine from ISHI. Designed in 1983 by and still available from Bill Cutler. Thinkfun's Blockout is a nice, portable, inexpensive copy. Awarded the Grand Prize at the 1986 Hikimi Wooden Puzzle Competition. |
 Three Pins By Jean Claude Constantin. Fit the six pieces in two layers into the tray, aligning holes so that the three pins can be inserted, each through two pieces. |
|

 Pack 6 - Eric Fuller Entered in the IPP 2003 Design Competition. |
 Sandwich - Vaclav Obsivac |
 HCP1 - Vaclav Obsivac |
|
 Malaga Box - Philos By Markus Goetz. |

Barrel Puzzle From Brian Menold at Wood Wonders |
 Stack the disks to form a cob. This seems to be a copy of the Toyo Glass puzzle "A-Maize-Ing." |
 Booze Crate |


Something Fishy also in wood - Fisherman's Dilemma, from Creative Crafthouse |

6-piece packing (Krasnoukov?) - from Rick Eason |
 Boxed LUV Stewart Coffin #189 a cheap Asian copy, but functional |
|
 A nice little packing puzzle handmade in the Ukraine. Purchased off eBay. I'm not sure, but I think this is the same puzzle as shown on www.golovolomki.ru in the Wooden Puzzles section, called "Disobedient Particles" by I.A. Nowitschkowa. |
 Russian 3-piece packing Obtained from Rick Eason at NYPP 2008. The label is in Cyrillic and I cannot read it! I'm not sure, but I think this is the same puzzle as shown on www.golovolomki.ru in the Wooden Puzzles section, called "Pythagorean Trousers 2" by I.A. Nowitschkowa. |
||
 Dice Box - Sticks |
 Dice Box - Prisms |
 Trevor Wood's Cube the Square - unknown craftsman The 8 pieces form a 4x4x4 or an 8x8x1. |
 Nob's LL Puzzle - unknown craftsman Each of the 8 pieces is made from two L tricubes. They pack a 3x4x4 box, made from purpleheart. |
 This is Packman by Gary Foshee. Get all of the elements into the cube so that all of its surfaces are flush. (Photo from John Rausch's site.) |

Meiji Apollo Fit the plastic candy replicas into the box in two layers. Purchased from Torito |
 Pack six pieces in the box, from Japan - purchased at IPP32 (I don't know the name or the designer) |
|
I found this interesting small Six Piece Cube Puzzle purportedly from Pussy of Germany though it has no markings or provenance. |
|||

Cat Stax - issued by Brainwright |

Dog Pile - Brainwright |
||
 Oskar van Deventer's Two-Piece Packing From Bernhard Schweitzer at NYPP 2008 |
 Quadron by Naef (1987) Designed by Jost Hanny |
 Fragmented Cube - Oskar van Deventer Pack eight pieces into the box. They can be packed such that faces appear with and without "holes." Purchased from Oskar at IPP28 in Prague. |
 Magellan - Philos Designed by Georg Pfaffinger 12 pieces pack into the 4x4x4 box and leave a 2x2x2 hole in the center. Includes other challenges. Purchased at a puzzle store in Prague. |
 Caboose, designed, made, and exchanged at IPP32 by Henry Strout |
 Nine Parts Packing, designed by David Goodman, made and exchanged at IPP32 by Dor Tietz |
 Six Cushion Shot, designed and made by Wayne Daniel, exchanged at IPP32 by Marti Reis |
 Tetracubed, designed by Robert Reid and George Miller, made by Wayne Daniel, exchanged at IPP32 by Stan Isaacs |


Hikimi "For Your Own Sake" 2-1 A six-piece "squashed" variant of Conway's Curious Cube - contains two voids when solved. |

Super Box 2, designed by J.C. Constantin. Pack the six pieces in the tray so none stick out. The dimensions are such that several arrangements seem like they might fit if you just force the pieces a wee bit - but do not! The solution does not require any forcing at all. |

P-26 Drehcube, designed by J.C. Constantin. Arrange the six pieces in the tray and form a 3x3x3 cube. Each piece is composed of four unit cubes and a half-cube triangular prism. On five of the six pieces, the triangular unit can be rotated in place to add to the confusion. |
|
 IQ Fit from
Smart Games.
IQ Fit from
Smart Games.
Designed by Raf Peeters. I found this at a Barnes & Noble store near me. |


Back2Back - designed by Raf Peeters, issued by Smart Games Place the pieces into the board, using both sides of the board. A polyomino-type packing puzzle, but with an added dimension - the pieces have some units that push through the grid and occupy spots on both sides, and others that occupy a spot on only one side, allowing another piece to overlap on the other side of the board. |

I found the IQ Steps in a local Barnes and Noble. It is another puzzle designed by the incomparable Raf Peeters and issued by Smart Games. |
|


John Hache posted a link to a vendor (Otakumode.com - also available elsewhere) selling these Nyanko Clay Pot Puzzles. Pack six different cat-shaped pieces into a bowl so the lid fits properly. I bought the versions with black cats (Kuroneko) and white cats (Mikeneko). There is a third version with tan cats (Doraneko). |
|||

Nine Blocks Box - designed by Jerry Loo, made by Eric Fuller from Holly, Zebrawood, and Macassar Ebony Pack the nine blocks in the box. Thoughtful magnetic closure and extra compartment for transporting unsolved. A fun little design I was able to solve with a bit of logic! |

LooZ Fit - designed by Laszlo Molnar made by Brian Menold from Koa (tray) and mixed woods |
||



Flooring - designed by Osanori Yamamoto, made by Tom Lensch Fit all five pieces into each well, always creating a flat solid "floor." |
|||


Two Packing Puzzle - from Jeff Baz. |


Three Packing Puzzle - from Jeff Baz. |
||




Broken Box - by Lucie Pauwels, made by Pelikan from Cherry and Wenge woods. The two pieces of the box can be magnetically attached in six different configurations. In every case, fit the nine pieces to fill the resulting contained space. |
|||









 Packed in Tokyo I got this in Japan. |
 Java Tea [A] |
 Packing Peanuts [B] |
|
Shot You |
 On the Rocks [A] |
 Pack the Asparagus Designed by Nob Yoshigahara Related to Tridiamonds |
 Pack the Beans [B] (I don't have this one.) |
 Pineapple Delight [A] [B] Related to Pentominoes |
 Pack the Pudding (or Custard) [B] |
 Pack the Beer [B] |
 Pack the Plums [A] [B] |
 Pack the Peanuts [B] |
 A-Maize-Ing [A] [B] |
 Pack the Rice Crackers [A] [B] |
 Pack the Orange [A] (I don't have this one.) |

 Home Alone Husband |
 Bin Cross [A] |
|

|
Enumerating Polyforms
Sloan's sequences given for: # free . # 1-sided (holes allowed) Wolfram links at top show initial pieces; links in table to Wolfram, Ishino's site, etc. show all pieces |
|||||
| n, prefix | -iamonds #A000577 .#A006534 Wolfram |
-ominoes #A000105 .#A000988 Wolfram |
-hexes #A000228 .#A006535 Wolfram Wikipedia |
-aboloes (-tans) #A006074 Wolfram Esser |
-cubes #A038119 .#A000162 Wolfram |
| 2 d[i]- |
1.1

|
1.1 | 1.1 | 3 | 1.1 |
|
Dick Hess designed
a puzzle using nine
planar tridiamonds.
The Naef Favus puzzle pieces are a set of planar and non-planar solid tri-diamond prisms. (Labeled dominoes are discussed in the Pattern section.) |
|||||
| 3 tr[i]- |
1.1 | 2.2 | 3.3 | 4 | 2.2 |
| The two triominoes consist of one three-in-a-row and one "L" - the L is non-convex. | |||||
| 4 tetr[a]- |
3.4 | 5.7

|
7.10


|
14.22

|
7.8


|
|
Tetromino sets: Tenyo BtC #783
See my diagram of polyhexagons up to tetrahexes. Naef's Hexagon puzzle uses the set of 7 free tetrahexes, made from metal nuts. The Snowflake puzzle by Stewart Coffin uses the set of three trihexes and seven tetrahexes. Michael Keller shows some figures and solutions made with the set of tetratans. The Eternity Delta puzzle is a commercial set of 14 tetratans. Kadon's Tan Tricks I includes 2 monotans, 3 ditans, and the 14 tetratans. Jurgen Koeller discusses tetracubes. The eight tetracubes are named: I O L T N, tower-right, tower-left, and tripod. They can make two boxes: 2x4x4 (1390 solutions) and 2x2x8. A set called Wit's End was produced by Lowe in 1967. Piet Hein's famous Soma cube uses the six non-convex tetracubes plus the single non-convex tricube. |
|||||
| 5 pent[a]- |
4.6

|
12.18

|
22.33



|
30.56 | 23.29 12 planar 17 non-p 

|
|
Ishino's page on pentiamonds.
Peri Spiele (Austria) makes a set of 19 n-iamond pieces packed into a Star-of-David tray. The set includes two tetriamonds, seven pentiamonds (all 4 possible + dups), six hexiamonds, three heptiamonds, and one octiamond. The 12 planar pentomino pieces are named by convention after the letters they resemble: F I L N P T U V W X Y Z. There are too many commercial pentomino sets to mention. Ishino's page on pentahexes. Commercial sets of pentahexes: Tenyo BtC #22, Hi-Q Fusion, Hi-Q Confusion Kadon's Tan Tricks II includes the set of 30 pentatans. There are 29 pentacubes - 12 planar (corresponding to and named like the pentominoes) and 17 non-planar. Stewart Coffin on solid pentominoes; Stewart Coffin's Unhappy Childhood puzzle Kadon's page naming the planar pentacubes; Kadon's page naming the non-planar pentacubes |
|||||
| 6 hex[a]- |
12.19

|
35.60


|
82.147 | 107 | 112.166 |
|
Ishino's page on hexiamonds.
Hexiamond sets: Tenyo BtC #6 Hexomino sets: Tenyo BtC #600, Spear's Multipuzzle George Miller sold a set of 82 hexahexes. Kadon's Tan Tricks III includes the set of 107 hextans. Kadon sells a set of 166 hexacubes. Livio Zucca's Sexehexes (not polyforms) |
|||||
| 7 hept[a]- sept[a]- |
24.43

|
108.196 | 333.620 | 318 | 607.1023 |
|
Ishino's page on heptiamonds.
Heptiamond sets: Tenyo BtC #24 Kadon sells a set of 108 heptominoes. Peter Esser's page of the 108 heptominoes. |
|||||
| 8 oct[a]- |
66.120 | 369.704 | 1448.2821 | 1116 | 3811.6922 |
|
Kadon sells a set of 66 octiamonds.
Ed Pegg Jr.'s page on octiamonds. Kadon sells a set of 369 octominoes. |
|||||
| 9 non[a]- enne[a]- |
160.307 | 1285 .2500 |
6572 .12942 |
3743 | 25413 .48311 |
| George Miller sold a set of 160 noniamonds. | |||||
| 10 dec[a]- |
448.866 | 4655 .9189 |
30490 .60639 |
13240 | 178083 .346543 |
| 11 endec[a]- |
1186 .2336 |
17073 .33896 |
143552 .286190 |
46476 | 1,279,537 .2,522,522 |
| 12 dodec[a]- |
3334 .6588 |
63600 .126759 |
683101 .1364621 |
9,371,094 .18,598,427 |
|
 Concept 5 |
 Yasumi |
 University Games Pentomino Set |
 Logika |


Kohner Hexed (thick and thin versions, and alternate cover) |
|



Choc-a-Bloc - from Kidult A Pentomino set - the 12 pieces resemble chocolate bar pieces and can be flipped over. Presented in a clear 6x10 case. |
||
| Pentomino sets made into games: | ||

Quintillions, a nice Pentominoes set, by Kadon. This product launched Kadon in 1979. |
||
 ZahlenLabyrinth - Logika |
 Camelot (castle pieces on top of flat pentominos - arrange the pieces to build the castle) |

 Springbok Pentominoes |
The 12 planar pentominoes can be fit into various rectangles:
|
The 12 planar solid pentacubes can be packed into various boxes:
|
Here is one of the 3x4x5 solutions, in case you need to put your set back in its box...
I I I I I X F N L L Y Y Y Y T X V V V T X F N L T X F Y Z T U F N V P X F N L P U Z Z Z T U W N V P U W W L P U Z W W P |


Checkerbox - Bill Cutler 12 checkered pentominoes pack into a 3x4x5 box |


Wit's End by Lowe from 1967 is a set of tetracubes. The instruction sheet gives several construction problems. |
 The Spear's Multipuzzle is a plastic set of hexominoes. It includes all 35 "free" hexominoes and duplicates of 7 of them. The pieces are essentially 2D - they are not built from unit cubes and cannot be built into 3D structures. The set comes with a 6x10 tray and a booklet of problems specifying subsets of pieces to be fit into the tray. |

Cubeit - a vintage polycube assembly puzzle from Peter Pan Playthings In its original package. |
|


 The Ten Yen puzzle, published in 1950 by the Multiple Products Corp. of NY, includes a monomino, domino, both trominoes, and 3 each of the tetrominoes and pentominoes. Kadon offers one. Pieces in three colors. One challenge is to create identical shapes from the sets of three different colored pieces. |


 A gift from Brett of three "Meiji Chocolate" plastic Polyomino puzzles by Hanayama - Milk (12 pentominoes), Black (11 hexominoes), and White (8 pieces) - find them at Kinokuniya. |

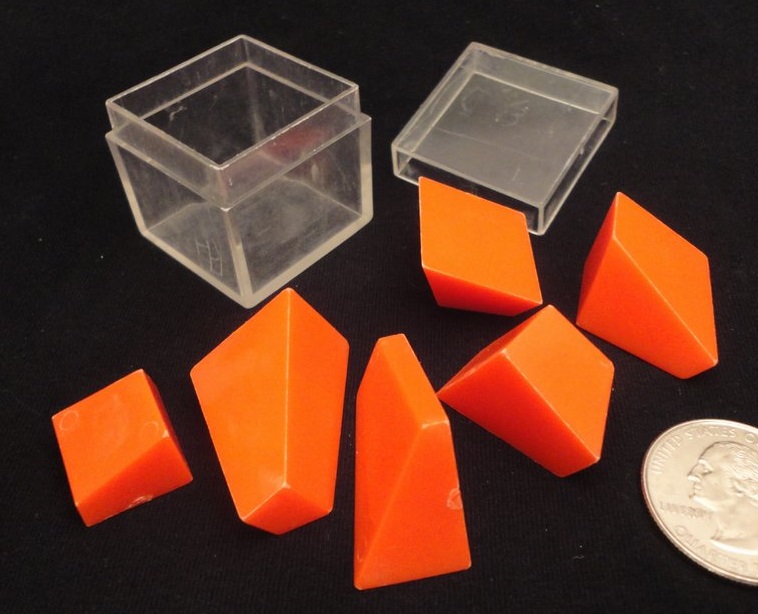
Cornucopia - designed by Peter Gal Thanks, Peter! |
|

3-4-5 iamonds - designed by Koshi Arai - IPP30 5 sets of the four pentiamonds. The 20 tiles will pack into both sides of the tray - the large triangle, and the double-layer diamond. |
|

I obtained a group of assorted polyform tray packing puzzles - some of which are duplicates of puzzles shown elsewhere, others of which are tangram and sliding piece puzzles. |


Peri Spiele (Austria) makes a set of 19 n-iamond pieces packed into a Star-of-David tray. The set includes two tetriamonds, seven pentiamonds (all 4 possible + dups), six hexiamonds, three heptiamonds, and one octiamond. I also found a set that says "Puzzle" instead of "Peri" in the black circle on the box. |
 A one-million pound prize was offered for the solution of the
Eternity Puzzle.
A one-million pound prize was offered for the solution of the
Eternity Puzzle.
I didn't win. The puzzle comprises 209 pieces called 12-polydrafters. For more info on the Eternity series, take a look at |
 The Eternity Delta puzzle was billed as a warm-up to the full Eternity.
It uses the set of 14 tetratans.
Here are some interesting sites discussing polytans:
The Eternity Delta puzzle was billed as a warm-up to the full Eternity.
It uses the set of 14 tetratans.
Here are some interesting sites discussing polytans:
|
 This is the Eternity Meteor puzzle. It uses a set of ten penta-hexagons. |
 Last but not least, the Eternity Heart. |
 I believe this is "Hextra" from Robert Longstaff Workshops. It uses a set of septa-hexagons. This is a gift from Carol Monica, the proprietress of one of the best puzzle shops around - the Games People Play shop in Cambridge, Mass. |
 The Snowflake puzzle was designed by Stewart Coffin (#3), and this version made of foam was offered by Binary Arts in 1993. It includes two sets of 3 tri-hexagons and 7 tetra-hexagons, a tray with two levels, and a booklet of challenges. |
 Here is an unnamed but colorful set of tetra-hexes in a clear case. |
 The "Hexagon Sense-A-Gone" is one in a series of Brain Drain puzzles from Mattel. It employs a set of 3 tri-hexagons and 7 tetra-hexagons. The pieces cannot be flipped, and only one of each of the pairs of mirror images is used. The pieces are prettily colored and suggest 3-dimensional cubes, but the instructions do not indicate any edge-matching constraint. Assemble them / Pack them in the tray. |
 Hexagon Sense-A-Gone Assembly |
 Profound Round Circle Dissection |
 Mangle Quadrangle Edge Matching |
 Checkle Heckle Checkerboard Dissection |
 Block Shock Edge Matching |
 Square Where Packing Equivalent to the Pressman Think Square puzzle. |
 Kadon Rombix |
 Galt Puzzle Blocks |
 TriPentaHexagon - George Miller |
||
|
||||

Plexi Roundominoes - issued by Brainwright A colorful set of 28 curvy shapes and a booklet of assembly challenges. Developed by Kadon. |

Plexi Iamondhex - issued by Brainwright A colorful set of 12 angular shapes and a booklet of assembly challenges. |
|||
|
Read about the Soma Cube on:
The Balanced Soma is an assembly such that the pieces remain together when balanced on a single cube placed at the center of the bottom face. At least six such constructions exist.
|
|||||||||||||||
|
|||||||||||||||

 The eight pieces of this Baumeisterspiel ("Master Builder") set from Logika include the Soma pieces, plus a 1x1x3. I also have a "mini" version with a handy cover. |
 Rhoma is like Soma, but with rhombic pieces. I have a large and a small Rhoma. |
 The Illusions from Magnif is similar to Rhoma. |
|||||||||||||
|
|||||||||||||||



Reticulated Cubes - designed by Lee Sallows, made by Eric Fuller from a variety of exotic woods including Black Limba, Walnut, Mahogany, Leopardwood, Wenge, Spalted Tamarind, Canarywood, Quilted Maple, Padauk, and Red Grandis (the box). A very nice execution of Sallows' puzzle - subsets of the nine pieces (three each of 8, 9, and 10 cubies) can be combined three at a time to form solid 3x3x3 cubes. Arrange the pieces in the 3x3 grid in the box so that every row, column, and main diagonal of three pieces can make a 3x3x3 cube. Sallows wrote a book about this puzzle called Geometric Magic Squares, published in 2013 by Dover. Eric has crafted a beautiful conversation piece here, and I enjoyed the logical process of solving this one. |
|||||||||||||||
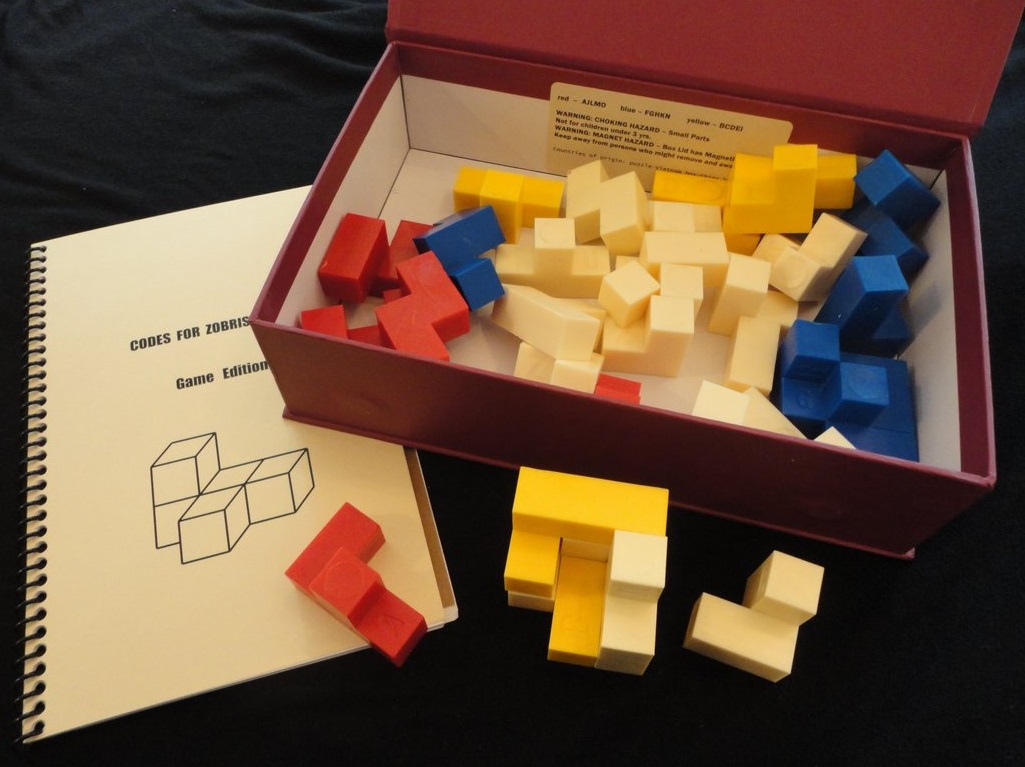
Zobrist Cube Set designed by Al Zobrist A nice magnetic-closure box containing 33 plastic pieces and a 56 page booklet specifying over 20,000 puzzles - each either a 3x3x3 cube, a 3x3x4 prism, or a 4x4x4 cube to be constructed from a subset of the pieces. Zobrist Cube Kickstarter www.zobristcube.com |
|||||||||||||||
 Bill Cutler's Splitting Headache yields a nice A-Ha moment when one solves it systematically. I think I bought this at Games of Berkeley many years ago. Discussed on Peter Kaldeway's site. May be available at Creative Crafthouse. |

Stewart Coffin's Half Hour Cube (#29) see the pieces at Puzzle Will Be Played... ; also see Chapter 3 in Puzzling World of Polyhedral Dissections (scroll down to Fig. 53) |
 The TetraCube Purchased from Wingstoys (defunct). Cheap Monkeypod wood. 13 pieces make a 4x4x4. One "L" tetracube, plus 12 pentacubes: 6 planar: F, L, P, T, W, Y, and 6 non-planar, 3 pairs of mirror images: (using Kadon's naming system) L1 and J1, L2 and J2, and L4 and J4. |
|||||||||||||

The Procrastinator Puzzle - designed and made by Andrew Crowell 20 Different Puzzles in One Set - Based on Stewart Coffin's Half Hour Puzzle |
|||||||||||||||
 The Bedlam Cube Wikipedia entry |


Bedlam Treasure Chest Gift from Brett. Thanks! |
 The Pedestal Problem has cubies joined at an offset, and must be assembled inside fenceposts |
|||||||||||||

The craftsman Scott T. Peterson of the state of Washington made this beautiful version of Stewart Coffin's Unhappy Childhood (#41) puzzle for me. Of the 17 non-planar solid pentominoes, 12 lack an axis of symmetry. Eliminate the two that fit into a 2x2x2 box to arrive at the ten pieces of this puzzle. Those ten pieces pack into a 2x5x5 box in 19,264 ways, and can be checkered in 512 ways. Only one of those possible checkerings has a unique solution (one other has no solution and the rest have multiple solutions) - this is the checkering for the Unhappy Childhood. I also obtained a version made by Jerry McFarland, called "Coffin's Cuboids." The diagram will help you pack your set into a 2x5x5 box, though it is not a solution since the arrangement shown won't make a proper checkerboard pattern. 
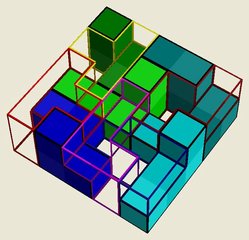
|
|||||||||||||||
 Cube from Melissa & Doug - the same set of planar pieces as the classic Diabolical Cube, which appears in Hoffmann's 1893 Puzzles Old and New. Also see Kevin Holmes' Compendium, page 3^3-3. |
 Metropolis |
 Rubik's Bricks |
|||||||||||||
|
|||||||||||||||

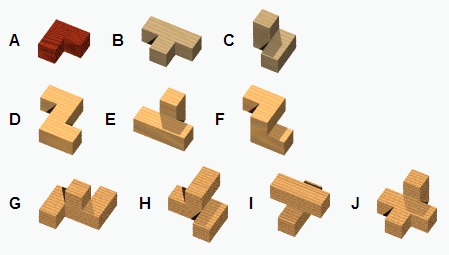
Naef Campanile Designed by Manfred Zipfel and Cordula von Tettau in 1979. See the Campanile pieces here. |


Professor Brain's Tower Puzzle 10 pieces, different from Campanile. |
 Here is a puzzle using pieces made from unit spheres - the pieces stack inside a cage. It is called "Cerebrum." |
|||||||||||||

 Flogik.de Skyscraper This is almost identical to Naef's Campanile (but made with much less quality). In the Skyscraper, piece 'B' has an extra cubie sticking up at the junction. |

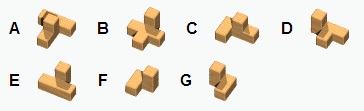
Tourelle - designed by Yavuz Demirhan, made by Brian Menold from Sheoak and Cocobolo Fit seven pieces into the frame - 1 solution. Similar idea to Campanile but there are no planar pieces and the entryway restricts piece orientations. |
 Double Cross (without the tray) (discontinued) from William Waite. Fit the 6 pieces together in 2 layers of 3. I think I actually prefer it without the tray - the pieces mate tightly and seem like they would be difficult to manipulate if they were in a tray. |
|||||||||||||
 Naef Escalon Designed by Jost Hanny. |
 Tetris Cube Designed by Matt Campbell, produced in 2007 by Imagination Games and tetris.com. 9839 solutions - confirmed by BurrTools. This is the small-sized cube. |
 Eclecticube - Kevin Holmes |
|||||||||||||
 Double Take - Mag Nif 2003 Eight pieces form a 4x4x4 cube or an 8x8 square. |
 Albertuv #4 The eight octacubes form a 4x4x4 cube or an 8x8x1. Purchased at a puzzle store in Prague. |
 Albertuv #8 The eight octacubes form a 4x4x4 cube or an 8x8x1. Purchased at a puzzle store in Prague. |
|||||||||||||
 KeshIQ erasers mfd by Seed Co. in Vietnam. Purchased from Eureka |
 Dollar Tree Hexagon Equiv. to Naef Favus at a fraction of the cost! (Favus was designed by Toshiaki Betsumiya.) |
 Japanese hexagon An Asian version of the Hexagon/Favus. |
|||||||||||||

 Werkstattwürfel 1 Designed by Bernhard Schweitzer |

The Question Mark Puzzle from Pentangle - six pieces form a cube in two ways, and also fit into the 3x6 box to form a question mark shape. - This is equivalent to the Steinhaus (aka Mikusinski's) Cube. |

Cube Conundrum from House of Marbles Purchased at the Vermont Toy Museum in Quechee Gorge Village. |
|||||||||||||


Pine Six-Piece Cube Provenance unknown. |
|||||||||||||||

Rubik's Puzzle - MegaHouse 2010 Nine planar polycube pieces, stickered using standard Rubik's colors; also a clear cubic container, and instruction sheet (in Japanese). Includes an "official" 1x1x1. Also 2 1x1x2, 3 tricubes, 2 tetracubes, and 1 pentacube (the 'F'). The pieces can be assembled into a 3x3x3 where the six faces are colored as a standard Rubik's Cube. |

4 Uni Cubes - idea by Marcel Gillen, Program by Georges Phillippe IPP18 (Tokyo) exchange puzzle from Luc De Smet Includes 7 plastic polycube pieces - the O, L, and T tetracubes, and four pentacubes - two mirror-image pairs N1 and N2, and S1 and S2. Comes packed in the box in a 2x4x4 arrangement. Five challenges - you can remove each of the four pentacube pieces in turn and with the remaining six pieces make a 3x3x3 cube; also, find an alternative to the 2x4x4 solid. |

A 7-piece cube by Galley Games. |
|||||||||||||


Akiyama Cube designed by Hisayoshi Akiyama. Made by Naoyuki Iwase (Osho). |
|||||||||||||||

Triple Triads - designed by D. Goodman and Abraham Jacob This was Abraham's exchange at IPP35. I really like this one - 3 copies each of simple tetracube pieces. Use the three copies of a given shape to construct a form, but construct the same form with each of the three triples! |
|||||||||||||||

Here are several "pyramid" puzzles that while each posessing only a few pieces, nonetheless can prove to be quite puzzling!
|
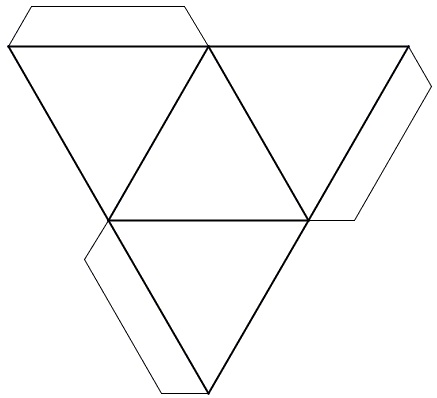
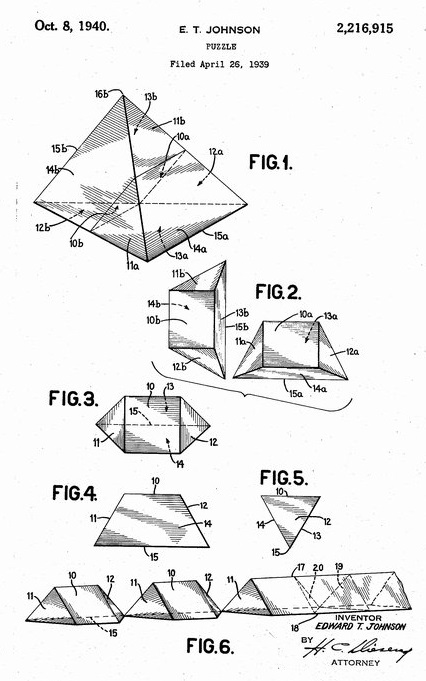
This is the classic two-piece tetrahedron patented by Edward T. Johnson in 1940
(U.S. Patent
2216915).
It was popularized in the following decade when a small plastic version became available from FUN Inc. of Chicago, starting in 1956, and has been produced ever since. The classic 2-piece pyramid has to be one of the most simple yet elegant puzzles devised. Once you've solved it, it gets old, but it is always fun to watch a newbie's first encounter with it! |
|
|||||||||||


|
||||||||||||
Many other examples have appeared - here are a few I have...


|
From the two-piece, things escalate...
Here are three examples of an interesting tetrahedron - it has three identical (and very pointy!) pieces.
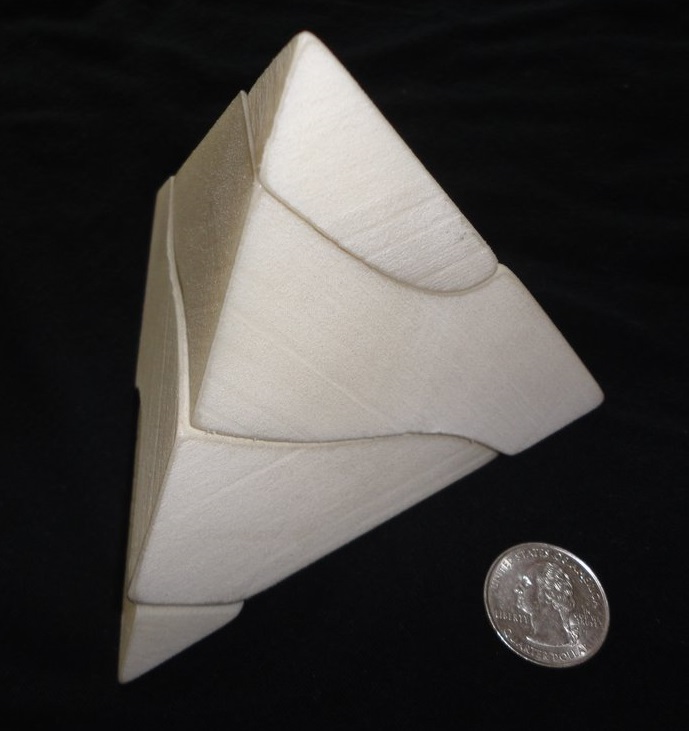
It has been called the Caltrops puzzle.


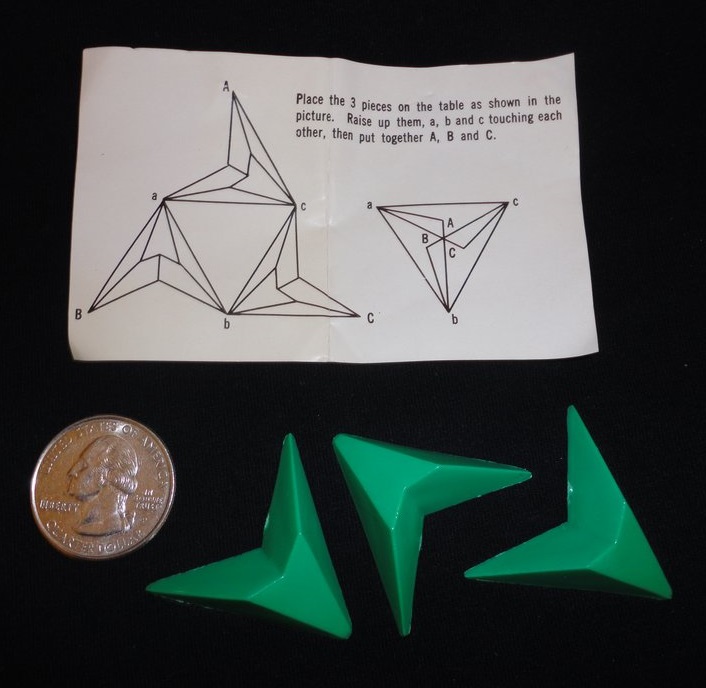
|
|||||||||||



Fire - designed by George Hart A two-piece dissection of a tetrahedron using a helical cut - this large but loose-fitting 3D printed example served for two months as a hands-on exhibit in a gallery at Stony Brook University. A gift from George - thanks! |
||||||||||||
|
||||||||||||


The Pyramidal Pile or Setting Hen suggested by Stewart Coffin, made by Brian Menold at Wood Wonders, from Holly and East Indian Rosewood. The units are truncated rhombic dodecahedra. See Stewart's discussion of puzzles made from rhombic dodecahedra . |
||||||||||||


Distorted Cube / Pyramid Pile designed by Stewart Coffin made by Brian Menold from Poplar Arrange the pieces to fill the box 3 different ways and also make two pyramids outside the box. |
||||||||||||

A [square] pyramidal pile. Each layer i, starting at the pinnacle, will have i2 balls in it. The entire pile will have (i * (i+1) * (2i+1))/6 balls in it - Thomas Harriot (1560-1621) seems to have been the first to figure out this equation. Here are the numbers of balls (with the total for the stack thus far shown in parens): 1 (1), 4 (5), 9 (14), 16 (30), 25 (55), 36 (91)... Note that the smallest pile which can be formed into both a square pyramid, and a flat square, has 24 layers and contains 4,900 balls (that can make a 70x70 flat square)! In 1918, G. N. Watson proved that there are no other solutions. |
See the diagram below -
the first layer of spheres is laid in a hexagonal arrangement.
The second layer goes into depressions formed by the first layer, but will only fit in one of two mutually exclusive subsets of the depressions.
Once we have placed the second layer, we now have a choice as to where to place the third layer.
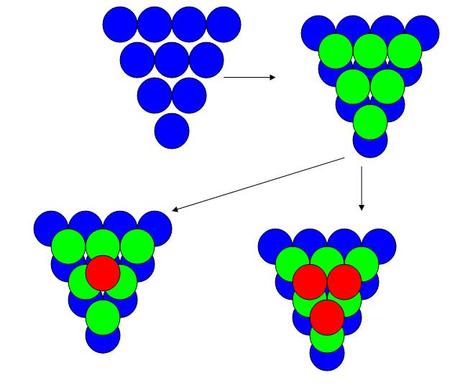 The lower-left-hand image shows an arrangement where the 3rd layer goes into depressions directly above spheres in the first layer.
The lower-right-hand image shows an arrangement where the 3rd layer goes into depressions above holes in the first layer.
The former is known as hexagonal close packing, and the latter is known as face centered cubic packing.
The face centered arrangement is used for Ball Pyramids and Tetrahedrons.
The lower-left-hand image shows an arrangement where the 3rd layer goes into depressions directly above spheres in the first layer.
The lower-right-hand image shows an arrangement where the 3rd layer goes into depressions above holes in the first layer.
The former is known as hexagonal close packing, and the latter is known as face centered cubic packing.
The face centered arrangement is used for Ball Pyramids and Tetrahedrons.
|

A tetrahedral pile. Each layer i, starting at the pinnacle, will have (i * (i+1))/2 balls in it (these are called the triangular numbers). A tetrahedron with i layers will have (i * (i+1) * (i+2))/6 balls in it (these are called the tetrahedral numbers - here are the numbers of balls (with the total for the stack thus far shown in parens): 1 (1), 3 (4), 6 (10), 10 (20), 15 (35)... |
|
Mag-nif and others have issued a classic ball pyramid (tetrahedron), comprising four pieces each composed of joined spheres. Mag-nif calls their version Tut's Tomb.
The German company Pussycat makes a diminutive equivalent version. 



|
Variations on the Tut's Tomb design (where the basic four pieces have been further divided) have appeared in plastic, metal, and wood...


|



A metal version from Bits & Pieces. The pieces are 2x 1x4, 4x 1x3. The House of Marbles Pyramid Puzzle is a wooden version of this (I don't have). |
||||||

A larger derivative called The Lost Game of the Pharaohs also has six pieces: 2x 1x6, 2x 2x5, 2x 3x4. (Pharaoh sculpture not included!) |
||||||||

Four Piece Ball Pyramid issued by Kinder Ferrero |
||||||||
|
||||||||
|
||||||||




Perplexing Pyramids - Gordon Bros. of Fair Oaks CA (c) 1976 item no. 154 Six pieces, equal to the Perplexing Pyramid from Gordon Bros. - but this package includes an additional challenge on the back. Use the six pieces to make what George Bell calls the "Roof" shape, with a 3x4 base. A timely find, as in the July 2014 issue #94 of Cubism for Fun, George Bell coincidentally has an article entitled Pyradox: A Pyramid Packing Paradox in which he analyzes sets of pieces that can form both a tetrahedron and a roof. 











|
||||||||

Pyradox - by George Bell. A nice set of challenges using five planar pieces and three boards. George's exchange gift at IPP38 San Diego. The roof shapes kicked my butt! |
||||||||
 Here is a 4-piece puzzle called "Der Fluch des Pharao" (Curse of the Pharaoh) by Markus Goetz, made by Philos and purchased from Funagain Games. The pieces actually do interlock but I still categorize this as an assembly rather than an interlocking puzzle. |
 Cubikon Ball Puzzle The pieces of the Ball Puzzle from Cubikon are all planar and have spheres joined at 90-degree angles. Contrast with the pieces of Fantastic Island which employ 60-degree joints. Fit the pieces in the tray, then use subsets of them to make pyramids. |

Kanoodle - SmartGames Fit the pieces in the tray, then use subsets of them to make pyramids. |
||||||

IQ Puzzler Pro - SmartGames A set of 12 planar poly-sphere pieces. The case provides both a rectangular grid and a diamond grid. Graduated planar challenges call for packing the pieces into both grids; another set of challenges entail building a side-5 pyramid. |
||||||||
 Puzzle in a Puzzle Box, designed, made, and exchanged at IPP32 by Thomas Beutner |
 Kolossal Pyramid from Kadon Designed by Len Gordon; 12 pieces (8 of one and 4 of another). 47 solutions. I don't have this. |


Two-piece Ball Tetrahedron - a 3D print from George Bell These two pieces do interlock and the tetrahedron snaps and holds together, but I have listed it here with other ball tetrahedron puzzles. |
||||||


The Bermuda Triangle, designed by Adrian Fisher and issued by Pentangle, is a five piece wooden tetrahedron. The pieces do not interlock, and there will be voids inside the completed puzzle. (Purchased at Cleverwood for $19.) |


The Tempil puzzle issued by Dalloz is a copy of the Bermuda Triangle. Purchased in auction from the John Ergatoudis collection. |

Packed Pyramid - designed and made by Bill Sheckels exchanged by Norton Starr at IPP35. 1. Build a pyramid from the four identical pieces. 2. Build it in the tray. 3. Pack the pieces within the tray. |
||||||||||



Packed Pyramid - Next Generation From Black Dog Puzzleworks (Bill Sheckels) |
||||||||||||



Pyrra was issued by Design Science Toys (defunct). It has 15 pieces and 3 distinct solutions. |
||||||||||||
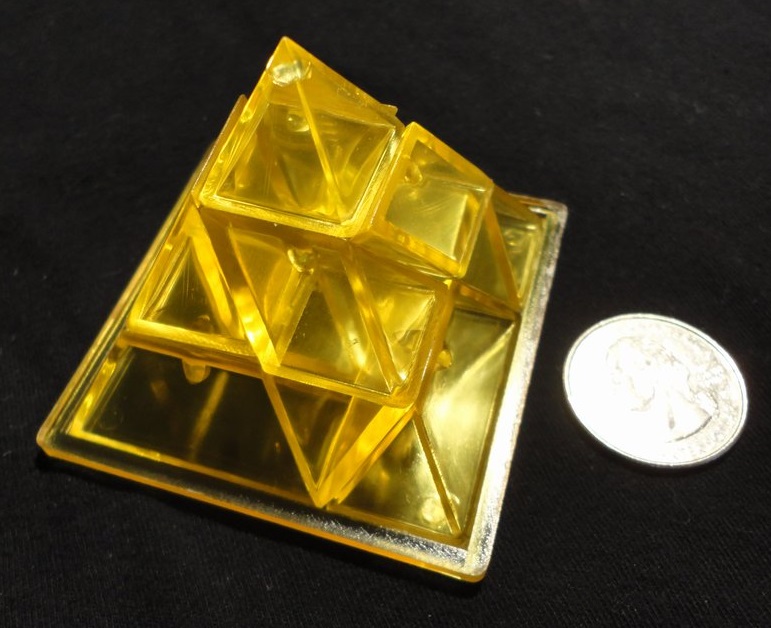

Dollar Tree Pyramid Equivalent to the Pyrra. |
||||||||||||


This is a ten-piece pyramid. No name or manufacturer info on the box, other than "Mindgame." Purchased at New England Hobby. There are at least two distinct solutions, since I found one by hand that is different from the supplied solution. The pieces are composed from two logical units - a square-based pyramid, and a tetrahedron (slightly stretched). There are a maximum of two tetrahedrons and 3 pyramids per piece. I like this one! |
||||||||||||





The "Bamboo" Pyramid has the same 10 pieces as the Mindgame pyramid shown above. The 10-piece has been offered under several brands. I don't have these. |
||||||||||||




Gizeh by Siebenstein Spiele - 8 pieces. I don't have this, but I would really like to find one! |
||||||||||||




This is a 9 piece pyramid. I don't have this. Creative Crafthouse offers a version. |
||||||||||||


Pieces of the 9-piece design can be combined into fewer pieces - above is a 5-piece pyramid. The 5-piece has also been offered with an attached cord - I have seen it called Khufu's Pyramid offered by Siam Mandalay. Each apex-forming piece has been combined with its adjoining tetrahedron, and pieces in the base are also simplified - two of the square-based pyramids have been combined with a tetrahedron to form the largest piece in the base. I don't have these. |
||||||||||||


Here are the Lupus, No. 5156 from Philos, in which the base has been further simplified to only two pieces, making a 4-piece pyramid, and the similar Choups from Dalloz. I don't have these. |
||||||||||||


Goki pyramid A five piece tetrahedron with irregular pieces. I don't have this. |
||||||||||||

I found this Pyramid Puzzle by Galley Games in a shop in St. Augustine Florida. It is the same as the Goki Pyramid. |
Blue RD Tetrahedron - advertising promo |
|||||||||||
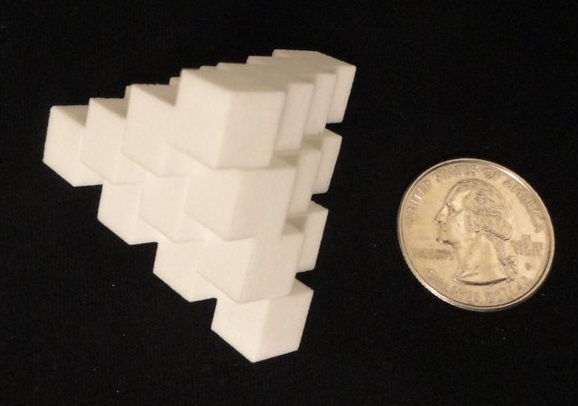
White Design Four Piece Pyramid - Bill Darrah Purchased from Bill's Shapeways shop. A nice compact challenging puzzle! |
||||||||||||

An Eraser Pyramid I don't have this. |



This is a 14 piece pyramid. Creative Crafthouse offers a version. I have seen it in various woods and different trays, also a version with a triangular collar piece that I suppose helps hold the assembly together. This has also appeared as the Luxor puzzle. I don't have this. |
|||||||||||


I got the PyrPlex from Andy Snowie. |

Philos offers a copy of Snowie's PyrPlex they call Gizeh (not to be confused with the Siebenstein Spiele version) - I don't have it. |
|||||||||||


This is the Pyramuddle. It seems to have been offered by Duncan Law circa 2011. I don't have this. |
||||||||||||
|
||||||||||||
 This is a Pyrix puzzle. Assemble a tetrahedron such that each face is a uniform color, constrained by the fixed threading of the pieces. U.S. Patent 5108100 - Essebaggers 1992 |
 From the same maker as Pyrix, Pyram consists of an octahedron and four smaller tetrahedrons, each having various patterns on their faces. Build a tetrahedron satisfying a pattern constraint. |

 The Pyrus Puzzle completes the three offered by Enpros. Like Pyram, an octahedron and four tetrahedrons. Build a larger tetrahedron having each of the four colors appear on every side. |
||||||||||
| The following puzzles, while advertised as "pyramids," are of course triangular prisms. | ||||||||||||


The 11 piece Beehive Pyramid. I don't have this. |

The 3 piece Pyramid. I don't have this. |
|||||||||||
There are various styles of dissection puzzle, but all of them involve some figure which has been cut up, or "dissected." The objective is usually to re-assemble the figure. Sometimes the pieces of a dissection are contrived such that an alternative figure can be assembled, too. In some cases, it is even possible to "hinge" the pieces to each other so that both forms can be assembled. See this link at Wolfram for more info on dissections.
 The Magic Square |

Square Up The pieces come arranged with a small square hole in the center - your task is to find a way to make a square containing no hole. |



Fourmost Puzzle - National Distillers A vintage advertising puzzle from the World's Fair. |
|



PM Whiskey Puzzle A nice large vintage cardboard advertising version of the square dissection devised in 1873 by Henri Perigal. |
|




Roddy Square Puzzle A nice vintage item from England. |
|
This design has been around for a while and has been called the Egyptian Puzzle. It is discussed on p.19 of Slocum and Botermans' "Puzzles Old & New." See U.S. Patent 907203 - Walker 1908
Typically the goal for this kind of dissection is to make a large square, not a rectangle (the rectangular shape is too easy). In the family of Egyptian-related puzzles, a given unit square, of which there are five, is usually divided into two or three pieces. Two pieces are formed when a unit square is sliced on a bias from a corner to the midpoint of an opposite side. The larger remainder can be further split into either a kite and small right triangle, or a large isosceles triangle and small right triangle, or three small right triangles. Dividing all 5 unit squares in two nets a ten-piece puzzle, the most common. Dividing one unit into 3 pieces nets an 11 piece puzzle. Dividing two unit squares in 3 nets a twelve-piece puzzle, etc. But the goal remains making a large square from the starting set of pieces whatever they may be. In addition, the pieces typically should not be flipped, and often advertising puzzles are printed on one side only.
Note the Dickinson's Seed Ten Card Puzzle - that one employs a novel dissection resulting in those two isosceles triangles - but it still arises from 5 unit squares, and the goal is still one big square. The Crestline Mystic Wedge goes to one extreme by dividing each of the five unit squares into four small right triangles.


|
|
 The Horse Blanket Puzzle was used as advertising for blankets made by Wm. Ayres & Sons of Philadelphia. |

 This twleve-piece version was used to advertise Devoe Paint. Note the kite-shaped pieces resulting from a couple of squares being doubly-sliced. |


 This cardboard version from 1943 is called "Bombing Mystery." |
|

Victory - a vintage 1943 cardboard Egyptian-type dissected square puzzle |
 Mystic Wedge by the Crestline Manufacturing Co.of Santa Ana CA. 20 equal right triangles (10 black, 10 red) make a square. Derive this one by first cutting each of the five squares in half into equal rectangles, then dividing each rectangle along a main diagonal. |


Dicksinson's Seed Ten Card Puzzle - 1910 Ten pieces with nice colorful graphics (blank on the reverse) must be arranged picture side up to form a square. Another variant of the Egyptian puzzle, similar to the Devoe 12-piece, but some right triangles have been fused to form two isoceles triangles. |
|
 The Tangram puzzle is a venerable classic where the real objective is to form various silhouettes from the given pieces. However, this version from Melissa & Doug is presented as a straightforward square-dissection and tray-packing problem. |
||
 Double Square - Thinkfun This is another fairly well-known design - form a square from 4 pieces, then add a fifth piece (a small square) to form another larger square. This design dates back at least as far as the 1934 Johnson Smith catalogue. |

 The St. Charles Milk Puzzle Seven pieces form a square. Discussed in Slocum and Botermans' The Book of Ingenious and Diabolical Puzzles on p.12. |
 Dickinson's Witch Hazel A vintage advertising promo. |
|
A vintage cardboard advertising puzzle - the 5-piece square - in its original envelope.
The product is Olympene, an "antiseptic liniment" produced by the patent medicine company Northrop and Lyman of Canada (ca. 1854 to 1980). Antique bottles of their elixers are collectible. (See an article at the University of New Brunswick.) Olympene seems to have been produced in the 1930s through the 50s. The puzzle was issued "compliments of Armstrong's Drug Store of Tillsonburg Ontario."
|
||
 The Elusive Square Puzzle - TSL Twelve pieces, whose collective area is 32 unit squares. What does that tell you about the solution? |
The Pythagorean Puzzle
Originally sold in London in the 1840s IPP30 exchange from James Dalgety Use the six pieces to prove the Pythagorean Theorem: For a right triangle, the sums of the squares on the sides equals the square on the hypotenuse. 
|
 Snider's Diamond Puzzle The 10 pieces form a square. Discussed in Slocum and Botermans New Book of Puzzles on p.14. |

Mensa's 8 Square Puzzle Made by China Industrial Ltd. Wow!Stuff. Starting with four of the eleven pieces, make a square. Then make squares with five of the pieces, six, etc. up to all eleven. |

Queijinho - form a square slice of cheese from the pieces. from Alexander Beresford (Dual Brain Games). Thanks, Alex! |
|

Mondrian Blocks - red set - mondrianblocks.com Manufacturer: Smart Egg Production & Licensing Given one of 88 starting positions for the 3 black pieces, position the rest in the 8x8 tray. There is no pattern challenge here, though each completed puzzle is reminiscent of a Mondrian painting. Nothing to do with this puzzle, but check out the Mondrian Puzzle on the YouTube channel Numberphile |
||
 Super Star - Melissa & Doug This is a dissection of a five-pointed star, in a tray. |
 Broken Heart Form a heart from the 9 pieces. |
 Doctor's Puzzle Board |
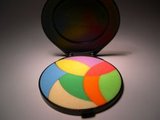 IQ Circle (PeToy Hong Kong) |
 Mind Bender Circle |
Squaring the Circle - Dollar Tree |
 Perfect Squares |
 Profound Round One of Mattel's Brain Drain series. |
 Fit the six pieces into the case to form a rectangle such that it contains only 3 straight seams. From puzzle-factory.com. |
 Form a six-pointed star using the six pieces. Also from puzzle-factory.com. |
 This set of "What's Your Score" puzzles from Shackman includes a dissected cross, square, and form a star. |
 Watney's Red Barrel puzzle Build a red barrel from the pieces. A nice symmetric dissection. |
 "Jeu de la Croix" is a vintage French boxed version of a dissected cross on a pedestal. |
 "La Cocotte" is a vintage French boxed puzzle - form a bird shape from eight isoceles right triangles. |
 Bibendum six-piece rectangle |
 "Jeu de l-Octogone" is a vintage French boxed dissection of an octogon into 12 pieces. (I don't have this.) |

The "Red Cross" or "Mysterious Cross" puzzle has been issued by several manufacturers of different nationalities and is known by various names. The eight red pieces form a Greek cross. The eight white triangles fill in the corners of the square. |
 IQ Mega-Form Circle |
 The Land Puzzle You are given a 2x2 square, with one corner unit square missing, leaving three unit squares. Cut the shape into four identical pieces. |
 Stacked Triangles - George Miller |
 Stacked Squares - George Miller |
 Flying Saucer, designed and exchanged at IPP32 by Jeremiah Farrell, made by Chris and Walt Hoppe |
 Nightmares, designed by Jeremiah Farrell, made by Walt Hoppe Would have been exchanged at IPP32 by Thomas Rogers (deceased) |

PEKE - designed by Kohfuh Satoh Form a Greek cross. made by Saul Bobroff at Here to There Puzzles of Beverly MA. a gift from Saul - Thanks! |

Greek Cross Puzzle - made by Bill Sheckels |
||
 





Spear's Shape Puzzles |
||

Squaring the Circle Jigsaw Puzzle - Copyright 1967 American Publishing Corp. Waltham, MA Not really a jigsaw, since the pieces do not interlock, and each pieces' edges do not uniquely identify its neighbors. Also not really "squaring the circle" - it simply comes with four identical curved pieces to be applied at the edges of a square, trivially making it into a circle. The challenges lies in forming a 9x9 square using the 11 polyomino pieces whose total area equals 81 units. |
||



DeLand's Circle Puzzle
Some time ago
I corresponded with Richard Kaufman
who had been researching the magician Theodore DeLand (1873-1931).
DeLand had created a vanish puzzle called La Mysterieuse in which I was interested -
I had found some
information on vanish puzzles in Richard's online publication
Genii Magazine.
(The thread cited references a nice online vanish puzzle called The Bermuda Square -
you can download a PDF here.)
In the course of our conversation Richard told me he
had found an illustration of DeLand's Circle Puzzle
and the only known set of instructions.
No physical copies (originally printed on celluloid) are known to exist.
Richard kindly gave me permission to re-create the puzzle and use it as my exchange at IPP35.
I worked with Pavel Curtis, who refined the design and laser-cut copies for me.
I include a four-piece border as an aid - the puzzle is more difficult if you at first try it without the border.

A vintage White Rose Tea Puzzle |
||
 Missing T - Thinkfun This is a version of the classic 'T' dissection, by Thinkfun. |
 Another classic T. |
 Pa's T from Drueke. |
 This cardboard version of the classic T dissection puzzle is a promotional item for a magician. |

 Chase & Sanborn Coffee-Tea Showing both sides of each of the four pieces, which form the usual T. |
 An H dissection puzzle was included in the vintage "Deluxe Puzzle Chest No. 3006" from F.A.O. Schwartz. |
 A political promotion - form the letters F and D. |
 The "Famous F" puzzle Note the trapezoidal piece - these pieces are pretty much the same as in the "FD Puzzle." |
 Cracker Jack F (I don't have this.) Similar to the "Famous F." |
 Fletcher's F - an advertising promotion. (I don't have this.) Different than the "Famous F." |
 Furnas - The New F Form an F from the six pieces. |
 Magic Z |

Dad's Boy K (I don't have this.) |

Kalamazoo K - produced by Mike Tanoff based on a vintage design called the "Dad's Boy Puzzle" Mike's exchange puzzle at IPP29 in San Francisco. Form the letter 'K' from the four pieces. |
|

An H Puzzle designed by Tomas Linden and made from Marblewood by Eric Fuller. |


LinkBelt M Puzzle |
|

Five-Piece K Dissection This one I consider not quite fair - the solution K is alright, but the cover and guide pictures are misrepresentative of the final shape. |

New H Puzzle - a vintage advertising letter dissection |
|

I was asked for help in solving The New B Puzzle from Gold Star Coffee. Seven pieces form a letter B. I don't have this, but I did figure out a solution. |


Form the word THINK from the 21 pieces. The pieces of each letter are easy to discern since the letter to which each piece belongs is embossed on its face. The T is the classic T dissection. The H is also familiar. The I is trivial. N and K gave some challenge. I also found a copy in its original package. |
|
 New*T, designed, made, and exchanged at IPP32 by Nick Baxter |


The vintage Celestial Cross puzzle issued by McLaughlin Bros. of NY. |
|



A vintage five-piece cardboard Number Nine Puzzle, issued by the National Carbon Division of Union Carbide and Carbon Corporation, advertising Eveready Batteries. I have obscured the borders of the individual pieces in the photo of the solution. |
||

 The vintage Red Cross Puzzle is a twelve-piece dissection of a cross (or the letter t).
The vintage Red Cross Puzzle is a twelve-piece dissection of a cross (or the letter t).
The small instructions slip identifies the source as The Gamo-Jig Company of 33 North Charter Street in Madison, Wisconsin. The pieces are heavy cardboard - the backs are plain, not red, so the pieces must not be flipped over. I have solved this one. |
 A dissection of a Rupee sign, designed and made by Scott Elliott |
|


Seven-to-One Puzzle (no date or provenance) Assemble seven pieces to form a mystery letter. |

Dissected A |
|

Another vintage advertising puzzle, from the Chicago Evening Post - form a letter Z from the six pieces. |
||

 Woodn't Tri - Reiss Form a square from the 4 pieces. Then form a triangle. This is a well-known dissection, originally called the "Haberdasher's Problem" and created in 1907 by Henry Dudeney. Discussed by Frederickson pp136-8. |
 Devil Puzzle This set of pieces can also be put together to form a rectangle. It was offered by Bits and Pieces. It was also offered as part of a series by Nob Yoshigahara. This is the same set of pieces as in the Anchor Kobold puzzle. |
 Dudeney's Zoo from Archimedes' Lab The triangle, pentagon, hexagon, and octagon are each dissected such that the pieces of each can form the square. 170mm x 120mm. |
 The Adams' Square and Cross. Form a square or Greek cross from the four pieces. (I don't have this.) |
Square and Cross - a tiny vintage Cracker Jack prize |
|

 Form a square or a Greek cross from the six pieces. An advertising premium from Molson - the pieces are nice 1/8" plastic. Note the similarity to the Adams Square or Cross - two pieces have simply been divided. |

"A Double Puzzle." A vintage advertising puzzle from Dickinson's. It is the same puzzle as the Molson Square or Cross puzzle. |
|
Mond oder Kreuz (Moon or Cross, aka Crescent or Cross) Make both a crescent moon, then a Greek cross from the pieces. From Wil Strijbos at IPP31 in Berlin. Thanks, Wil! A nice wooden version of Sam Loyd's Cross and Crescent dissection/transformation between the crescent and a Greek cross (plus sign). Notable because of the curved edges accommodated. Notice the flattening of the tips of the crescent. The nice 7-piece dissection shown was actually found by Harry Lindgren. It avoids thin slivers and differs from Loyd's solution. Discussed by Frederickson on pp167-9. |
||
 Cut Out Puzzle You are given a 2x3 rectangle, with one corner unit square missing, leaving five unit squares. Cut the shape into three pieces, which can be re-arranged to form a square. |
 Spade and Heart by Mineyuki Uyematsu Make a Spade or a Heart from the four pieces. Purchased at IPP28 in Prague. |
|


A vintage Cracker Jack premium - the "Chicken and the Egg Puzzle" |

T+3 - designed by Hiroshi Yamamoto The 3 pieces can be arranged to form four different pentominoes, including a T. A really nice dissection! This won a Jury Honorable Mention at the 2011 IPP Nob Yoshigahara Puzzle Design Competition. |
|


|
Over the years, there have been many variants on the theme of a dissected checker- or chess- board. Jacques Haubrich has published a compendium of checkerboard puzzles in two volumes. The first volume, "A Century of Checkerboard Puzzles," describes all known checkerboard puzzles - over 440 of 190 different types - published between 1880 and 1980. The second volume, "Additional Checkerboard Puzzle Designs," covers checkerboard puzzles published in the last 25 years. |
Jacques characterizes the puzzles using a code of the following format and meaning:
N[2].D.S-L
|


Slocum and Botermans, in their 1986 book "Puzzles Old & New" suggest that the first checkerboard puzzle was this Sectional Checkerboard of 15 pieces, patented in 1880 by Henry Luers (231963) and produced by Selchow and Righter. |



This "Krazee Checkerboard Puzzle" was made by The Plas-trix Co. Inc. Jamaica NY. There is no date on it, though in Haubrich's "Century" the date listed is 1957. This variation has code 12.11.3-7. My Dad had a puzzle like this, but it's gone - and I don't remember which variant it was. |

"The Famous Checkerboard Puzzle" - "Only 12 pieces but - Oh My!" |



This is the Bug House puzzle. Jacques gives a date of 1912. This has metal, rather than cardboard, pieces. It was offered in a rectangular box, and in a square box. It has code 14.14.3-5. |
 This one is the "Unique Original Checker Board Puzzle" from the Unique Novelty Company, and not only is it "Improved" but it is also the "Most Difficult Puzzle Known." It has code 14.14.3-5 and is the same set of pieces as the Bug House. No date is given in Jacques' book, but Slocum and Botermans bracket this in 1930-1940. |
 This one, "manufactured by J. F. Friedel Co., Syracuse, N.Y." calls itself "The Original Checkerboard Puzzle." I have no idea if the claim is true. There are 15 pieces and the price on the box says 10 cents. Jacques gives no date. Code is 15.14.3-6. |
 The Famous and Baffling Checker Board Puzzle has fourteen pieces and originally cost fifteen cents. 1927, code is 14.14.3-5. Inside the cover, the Vasen Mfg. Company of Davenport, Iowa, ran a contest offering $500 in Gold for the greatest number of correct solutions. Unfortunately, the contest expired July 15, 1928. |
 Checkle Heckle is a checkerboard dissection in the Mattel Brain Drain series from 1969. It consists of a tromino, 4 tetrominoes, and 9 pentominoes. The pieces cannot be flipped, so some mirror images are included. This is the same set of pieces as the Famous and Baffling Checker Board Puzzle. Code is 14.14.3-5. |
 Angle Mania has 15 pieces, but only 14 are needed to complete the puzzle. Four different pieces can be left unused. From 1984. Code: 15.15.2-6. |
 This is a recent wooden variation called just the "Chess Box." It includes a set of 12 checkered pentominoes plus a 2x2 checkered square piece. |
 Golf Tease - Great American Puzzle Factory 1996. Assemble 14 pieces into a 9x9 checkered square. |
 An advertising puzzle of 14 polyominoes from AMF. |

The older version of the TSL Draughtboard Puzzle. |

The newer version of the TSL Draughtboard Puzzle. |
 But - Oh My! |
 An advertising puzzle for the Burlington Railroad. |
 All Square |
 Uneasy Checkers |

Tenyo Checker puzzle |

Tenyo Checker puzzle (back) |

A vintage 12-piece Banzee Island Checkerboard Puzzle. An advertising promo for McCracken Realty Inc of Phoenix AZ. |

Sectional Checkerboard Puzzle - a vintage advertising promotion from the Phenyo-Caffein Co. of Worcseter MA. "Patented Sept. 7, 1880." This is the 15-piece Luers design. |

Phenyo-Caffein Checkerboard cont'd |
Phenyo-Caffein Checkerboard cont'd |

Check Saw - vintage checkerboard dissection from Shackman |

A vintage X-cel Checkerboard Puzzle |
|

|

Baffel - a vintage wooden checkerboard dissection in a tray. 17 pieces, including a 1x1 and two 1x2s. The pieces are stained on only one side and may not be flipped. Supplied with the original box cover and base but no box sides. 1939 A. C. Braden - Play Equipment Co. Los Angeles CA How about that sneaky gnome in the foreground, hiding a piece behind his back! |

|


|
There are several cubic puzzles in the form of a dissected die. In Hoffmann's Puzzles Old & New, The Spots Puzzle is number XVII in chapter III. The puzzle consists of nine 1x1x3 bars, each decorated with some pattern of spots (pips on the die). The task is to assemble a 3x3x3 replica of a die, having the correct arrangement of pips on all six sides. The modern puzzles below are all based on the same principle. | |
|


Intelligence Puzzler |
 Cracked Dice - Lakeside 1969 There are three dice - one whole (serves as a prototype) and the other two dissected into three 1x3x3 pieces each. |
 Make a Dice Puzzle Can you solve in 8 minutes? Copyright 1957 St. Pierre & Patterson Mfg. Co. |
 The Broken Die - Gantt's Wood Things |
 made in China |
|
 Twice Dice - Pentangle (small version) |
 Twice Dice - Pentangle (large version) |
 Woodn't Die - Mag-Nif |
 I found another dissected die - it seems fairly old - it comes in a purple box and has nine red pieces with white pips.
I found another dissected die - it seems fairly old - it comes in a purple box and has nine red pieces with white pips.
I am unsure of the provenance - there are no markings on the box or pieces. The box is 1.75"^3 (45mm^3). There should be at least 21 pips, but there are only 20 - so evidently one is missing. The pieces are not the same as the Wolff Spots Puzzle described in Hoffmann. |
||

A vintage Shackman "Dice" assembly puzzle, with instructions |

"Vegas Baby" cube from SiamMandalay (A gift from my brother.) |
|
 Symmetrick, designed by Vesa Timonen, made and exchanged at IPP32 by Tomas Linden |
 Balance of Power, designed, made, and exchanged at IPP32 by Rod Bogart |
 Symmetric Shape, designed, made, and exchanged at IPP32 by Emrehan Halici |

Trapezoid Symmetry - designed by Yasuhiro Hashimoto Form 3 symmetric figures using only the two smaller pieces. Then form 5 symmetric figures using all 3 pieces. |

Trick Symmetric - V. Krasnoukhov |

Bindi - V. Krasnoukhov |
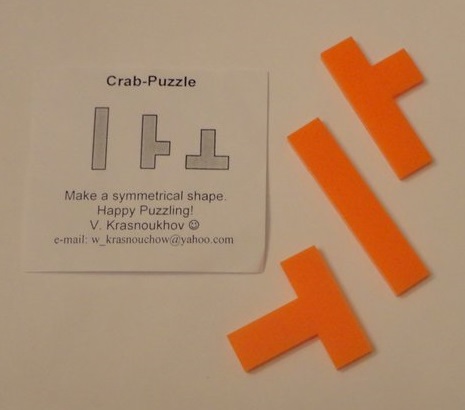
Crab Puzzle - V. Krasnoukhov |

C'est la Vie - Camden Lock |

Three Pentagons - Arai |

Symm-Aster - designed by Vladimir Krasnoukhov purchased from him at IPP35. Using the three pieces, make six symmetric shapes where the cut-out stars are also symmetrically arranged. |

Think Twice - designed by Vladimir Krasnoukhov purchased from him at IPP35. Using the three pieces, make two symmetric shapes. |

Tzr Shirt From PuzzleMist (William Waite) Arrange the six pieces to form a T-Shirt. A fair and pleasing solution. |

7-4-2 Make 2 crosses. From 1002 Puzzles (Lucie Pauwels) |

4 Huts 8 Huts - designed by Yoshiyuki Kotani Make the same shape from the 4 large huts, and from the 8 small huts. |

Secret Tricky Twins 1-3 - designed by Tadao Kitazawa, Vesa Timonen, Mineyuki Uyematsu Arrange each pair of like-colored pieces to make a polygon that can be divided into two identical polygons. I find these more difficult to approach and validate than symmetric-shape challenges. |

Unicum - designed by Norbert Galla, made by Brian Menold A gift from Brian at RPP2018 - Thanks! Arrange the three pieces to make a symmetric shape. |

Find Your P's Q's and T's - designed by Michael Dowle A gift from Allard at RPP2018 - Thanks! Arrange the three pieces to make several different triangles, quadrilaterals, and Pentagons. |

Spir ala rips - designed by Vesa Timonen, made by Tomas Linden for IPP37 A gift from John Rausch at RPP2018 - Thanks! Arrange the two pieces flat on the table so the resulting shape can be bisected into two identical shapes. |

Fraternal Twins - designed by George Sicherman and made by Brian Menold, from Spalted Alder and Benji. Assemble the two identical pieces such that they form a shape having mirror symmetry. |
||
|
A set of symmetric shape puzzles by Vladimir Krasnoukhov, produced by
Recent Toys
and purchased from my friends at PuzzleMaster.
|
||
|
Symmetry puzzles from
NothingYetDesigns:
These are all designed by Oleg Smol'Yakov:
|
||
 I bought an "8-block Collusion" puzzle from
Rocky Chiaro.
Rocky refers to the Collusion and its relatives as "pin puzzles."
I solved Rocky's Collusion and realized it was similar in principle to several other
puzzles in my collection such as Jean Claude Constantin's "The Fence"
that don't necessarily employ pins.
I call this group of puzzles the "Crossed Sticks Family."
A set of rods/sticks are crossed in two layers, with the points where each rod crosses (mates with) another
constrained by a feature present at that location on the rods, and the compatability of the respective features.
The crossings define a grid.
Identical overall physical dimensions make the rods interchangeable (except for their features), and features
are positioned at crossing points.
The notching positions are well-defined along the rod, and the number of potential notch positions is
related to how many rods cross.
When rods can be inserted only one direction into a frame, I call them "asymmetric."
I bought an "8-block Collusion" puzzle from
Rocky Chiaro.
Rocky refers to the Collusion and its relatives as "pin puzzles."
I solved Rocky's Collusion and realized it was similar in principle to several other
puzzles in my collection such as Jean Claude Constantin's "The Fence"
that don't necessarily employ pins.
I call this group of puzzles the "Crossed Sticks Family."
A set of rods/sticks are crossed in two layers, with the points where each rod crosses (mates with) another
constrained by a feature present at that location on the rods, and the compatability of the respective features.
The crossings define a grid.
Identical overall physical dimensions make the rods interchangeable (except for their features), and features
are positioned at crossing points.
The notching positions are well-defined along the rod, and the number of potential notch positions is
related to how many rods cross.
When rods can be inserted only one direction into a frame, I call them "asymmetric."
|



 The progenitor of this family seems to be this puzzle called Sputnik, made in the 1950s in Japan.
The progenitor of this family seems to be this puzzle called Sputnik, made in the 1950s in Japan.
There was also a version from 1958 with six sticks called the "Mysto-Peg Puzzle." Sputnik is described on page 59 of Jerry Slocum's and Jack Botermans' 1987 book "Puzzles Old and New." Rocky says it was his inspiration for his pin puzzles. |
| Two-Axis Arrangements, Two Features, 3x3 and 4x4, With a Frame | ||||
|
||||


Haba Crux Asymmetric Pieces: 0, 1, 2, 6, 12, 13, 14, 15 One solution. |
 A nice hefty lucite 4x4 weave puzzle - I found it in Montreal. Pieces: 0, 1, 2, 6, 12, 13, 14, 15. (Same as Haba Crux.) |
 This is a cheap monkeypod wood version called the "Snag Box," also known as the "Computer Chip." Pieces: 0, 1, 2, 3, 6, 7, 14, 15. Two solutions. |
||
 Here is another 4x4, called "Weaver's Dilemma." I don't own this puzzle, but I wanted to show it because it uses duplicates of several pieces. Pieces: 0, 2, 2, 3, 6, 6, 15, 15 |


A wooden 3x3 weave puzzle from "The Akron Los Angeles CA 90038." Made in Japan. Pieces: 0, 1, 1, 5, 5, 7. |
|||


The Fence Jean Claude Constantin Pieces: 1, 2, 3, 5, 6, 7, 9, 11. |
||||

IQ Stixx - SmartGames A set of sticks (2 feature type) along with a booklet of challenges. Raf told me the challenge in creating this was to select a set of sticks that could have many solutions, not just one or a few. |
||||
| Two-Axis Arrangements, Three Features, 3x3, 4x4, 5x5, No Frame | ||||
|
||||

Timbers by Mad Cow Pieces: 010, 120, 200, 201, 202, 220. |
The Log Pile puzzle uses 10 sticks in 2 crossed layers of 5. There are 13 pegs and 13 holes, so no hole/flat or hole/hole matings will be possible. Pieces: 00110, 01012, 02011, 02101, 02121, 10121, 11012, 11202, 11212, 12112. |
|||
| Three-Axis Arrangements | ||||
 The "Clive Cube" is a representative of this group, extending it from the 2 axes employed above, to 3 orthogonal axes. |

 The "IQ Puzzle" or "Ten Pins" puzzle is another 3D example. |
 I got this version from Torito. They call it "Sapience Sticks." |
||
 The Nine of Swords |
 This is the plastic Reiss version of the Nine of Swords. |
 Arjeu Achille CT5152 Purchased at GPP. |
||
| Special Arrangements | ||||
 Haba Verticus - designed by Heinz Meister Ten sticks, with holes or flats at 5 positions. Arrange them in two crossed layers of 5 each, such that holes in both layers all align. |
 Rick Eason's Keyhole Puzzle calls for 6 sticks to be arranged in a 3x3 sandwich, but with the additional complexity of sequential assembly. The pegs are screws and the holes are "keyholes" into which the screwheads must slide in the proper direction. |
 Rick has taken the keyhole concept into a new dimension with his Keyhole Cube. |
||

Prismazul Octuple - designed by Ingo Uhl, made by Logika Spiele Exchanged at IPP31 in Berlin by Tanya Thompson, purchased from Tanya. Build a triangular prism with the eight pieces - 4 equilateral triangles each with a footprint of 4 unit triangles, and 4 rhombuses each with a footprint of 4 triangles. Each piece can have unit triangles at three different levels: L, M, and H. There are 11 unit triangles at L, 10 at M, and 11 at H. The rhombuses preclude the target shape being a prism like a Toblerone bar, with a cross-section of an edge-2 triangle. A triangular prism with a cross-section of an edge-3 triangle is also precluded since the available 32 unit triangles could not be distributed evenly. (Each layer would have a footprint of 9 triangles - 3 layers uses 27, which is too few, and 4 layers uses 36, which is too many.) That leaves a 2-layer triangular prism with a cross-section of an edge-4 triangle, giving a layer footprint of 16 unit triangles. This implies that the pieces be arranged in two layers each containing 2 triangles and 2 rhombuses. (The four rhombuses alone cannot tile a side-4 triangle, neither can one rhombus with 3 triangular pieces.) If the pieces in the layers are oriented so that their multi-level faces face the opposite layer, then the 10 M units will mate 5x5, and the 11 L's will mate with the 11 H's. The indication that there should be 5 M units in each layer seems like a good clue! |
||||


Alexandre Muñiz contacted me regarding "crossed stick" puzzles. He has designed several, and very kindly sent me examples of his 10-piece Decagram, and his 8-piece 4-Pointed Star. You can read more at his website PuzzleZapper.com. Thanks, Ali! |
||||
|
Most of the puzzles in my "Weave or Crossed Sticks" family belong in the Assembly category because the layers of pieces simply lay one atop another - they do not interlace over and under. However, the pieces in the lattice structures below do interlace over and under - perhaps I should move them to the Interlocking category.
|
||||

Partitions - designed by Goh Pit Khiam, made to order from stainless steel and purchashed from Jerry Loo at JL Puzzles. |
||||
Collusion
|
The Fence
|
Timbers, Log Pile
|
Stabpuzzle
|
Rob's Magnetic Puzzle
|


 Prairie-Dog Town, Alien Hive, and Tee Time
each include 6 pieces (they're really the same puzzle)
that must be arranged in a 3x3 "sandwich" between two 3x3-holed plates.
Prarie-Dog Town is fun to analyze and solve.
These puzzles are slightly different from the previous examples, in that a piece can have "pegs" (or bumps)
on both sides.
The puzzle uses six planks of width 1 and length 3 units, and includes two 3x3 plates with all holes.
At each of the 3 positions on a side of a plank, there may be
a hole (which goes through the plank and therefore also appears on the other side in the same position),
a flat, or a peg (which fits into a hole).
When the pieces are mated, a peg must mate with a hole from one side, and this blocks BOTH sides of the hole.
Two holes, two flats, or one of each may also mate.
So a length-3 plank has 6 positions at which a hole, flat, or peg could exist.
For any plank, there may be a maximum of 3 holes or 6 pegs.
Below is my enumeration of all possible pieces for this style of puzzle.
The Prairie-Dog Town puzzle utilizes 6 pieces,
outlined in red in the chart (the piece with 2 bumps and 1 hole, with the hole on an end, is used twice).
I have shaded green the cells containing only pieces having at least one pair of opposing pegs.
Prairie-Dog Town, Alien Hive, and Tee Time
each include 6 pieces (they're really the same puzzle)
that must be arranged in a 3x3 "sandwich" between two 3x3-holed plates.
Prarie-Dog Town is fun to analyze and solve.
These puzzles are slightly different from the previous examples, in that a piece can have "pegs" (or bumps)
on both sides.
The puzzle uses six planks of width 1 and length 3 units, and includes two 3x3 plates with all holes.
At each of the 3 positions on a side of a plank, there may be
a hole (which goes through the plank and therefore also appears on the other side in the same position),
a flat, or a peg (which fits into a hole).
When the pieces are mated, a peg must mate with a hole from one side, and this blocks BOTH sides of the hole.
Two holes, two flats, or one of each may also mate.
So a length-3 plank has 6 positions at which a hole, flat, or peg could exist.
For any plank, there may be a maximum of 3 holes or 6 pegs.
Below is my enumeration of all possible pieces for this style of puzzle.
The Prairie-Dog Town puzzle utilizes 6 pieces,
outlined in red in the chart (the piece with 2 bumps and 1 hole, with the hole on an end, is used twice).
I have shaded green the cells containing only pieces having at least one pair of opposing pegs.
I have added this category because there are several puzzles that one can argue belong in the assembly section, since they are neither true interlocking puzzles, nor are they true sequential motion puzzles where long operators are needed, yet they do require some back-and-forth or rotational movement of the pieces, and the order of placement of the pieces can matter.
 Crossroad - designed by Goh Pit Khiam and made by Walter Hoppe. Purchased from Walter at IPP28 in Prague. |
 Pack the Square, designed by Goh Pit Khiam, made by Joe Sarabande, exchanged at IPP32 by Larry Seidman |
 The ODD Puzzle - designed by Hirokazu Iwasawa (Iwahiro). Three pieces (two identical) to pack into the box. Winner of the "Puzzle of the Year" Award in the IPP28 Design Competition. |
|
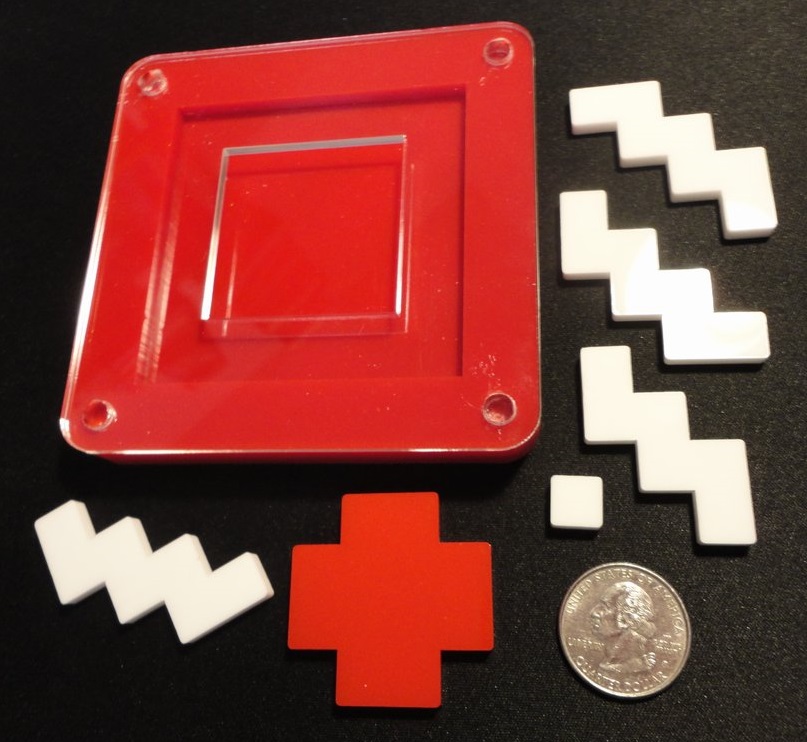
Heat Wave - designed by Goh Pit Khiam, issued at IPP35 in Ottawa. Fit the pieces in the tray, through the restricted opening. |
|||



Slide Swipe - designed by Haym Hirsh, made by Brian Menold at Wood Wonders, from Lacewood. This example has a piece storage compartment on the bottom, accessed via a magnetic lid. Fit different prescribed subsets of pieces (all flat polyominoes) into a single 5x5 layer in the tray, through a centered 3x3 opening. |
|||

ODD - designed by Hirokazu Iwasawa Made by Eric Fuller from Bird's Eye Maple and Walnut |


Wandering Cubes - designed by Peter Gal Made by Eric Fuller from Carolina Ash and Canarywood |
||
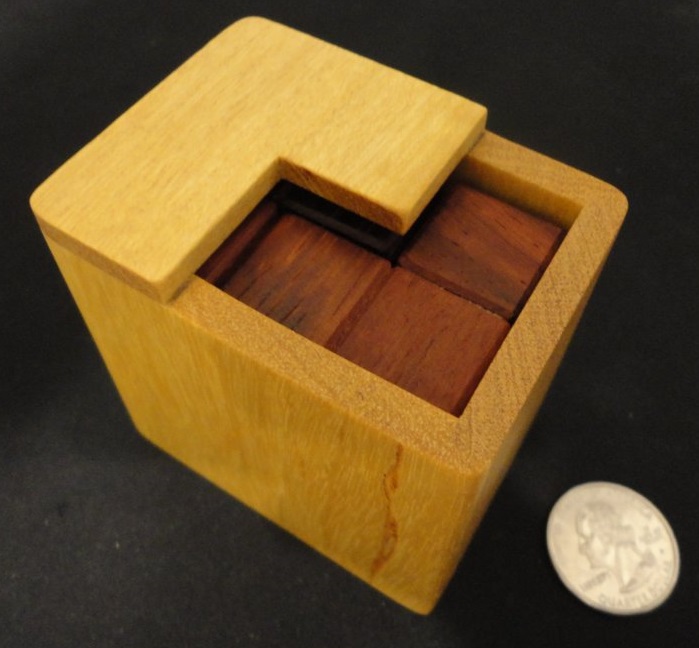


Kamelle Box - designed by Christoph Lohe, made by Brian Menold March 2018 Sequentially pack the three pieces into the 2x3x3 box through the restricted opening. The bottom is acrylic and has a useful opening. |
|||

Soma Tube - designed by Laszlo Molnar, made by Brian Menold, purchased at RPP in 2018 Sequentially pack the Soma pieces into the Kingwood box through the restricted opening. The box contains one fixed cubie. |
|||
 Eight Pack - designed by Tom Jolly and issued by Philos Pack eight tetracubes (four tower-left and four tower-right) into a 4x4x4 cage. Purchased from a puzzle store in Prague. |

Here is a set of pieces in a cage. I received this puzzle in a trade with P. F. Ramos - he designed it and Interlocking Puzzles (now defunct) made it. It is called Twin Pentominoes Into a Light Box. There are two instances of each of the non-planar pentomino pieces. |

Pentominoes in Cage from Brian Menold at Wood Wonders |
|

 Closterman cube Six pieces fit sequentially into a cubic cage. Nicely handmade in Yellowheart wood. |

Mine's Cube in Cage from Brian Menold at Wood Wonders |

Framework II - designed by Markus Götz Made by Eric Fuller, from Walnut, Sapele, and various other exotic woods. A redesign by Markus of Markus' original, with analysis by Tom Jolly. This version has a single solution. The pieces fit into the tray which is open on both sides. Offset tabs attached to the piece blocks grip both the frame and other pieces, making this somewhat interlocking and requiring sequential [dis]assembly. |
|


Mondrian's Square - designed by Tom Jolly made by CubicDissection |
|||
 Brunnenspiel, by Markus Goetz |

 Circelei - Hendrik Haak IPP26 Fit three hinged 3-layer polyominoes into three stacked trays. |


This is the Lolly Box, designed by Alfons Eyckmans and made by Eric Fuller - a walnut box, with Bubinga, Pau Ferro, Purpleheart, and Paduak pieces. |
|

Lolly Box 2 - designed and made by Alfons Eyckmans from Walnut and various woods Level 5.29 |

Four in a Box designed by, made by, and purchased from Alfons Eyckmans |

Lolly Box, Lolly Box 2, and Four in a Box - all designed by Alfons Eyckmans |
|


Bramble Box - designed by Noah Prettyman, made by Eric Fuller from Black Limba with pieces from Maple, Cherry, Sapele and Wenge. Four pieces in a box, level 19.4. |
|||

On 6/29/13 I had fun attending a puzzle party hosted by Saul and Paulette Bobroff at their home. Several notable puzzlers were there and we all got to play with much of Saul's extensive puzzle collection. Saul kindly gave me one of his IPP31 exchange puzzles - design number 138A by Stewart Coffin - the Piggy Box. Thanks, Saul and Paulette - I had a great time! |

Packuliar designed by Tom Jolly made by Brian Menold Pack the four pieces into the box - a sequential assembly puzzle. No solved image - too much of a hint. |


Mini Chessboard - designed by William Hu Made by Eric Fuller from Peruvian Walnut and Maple |
|

In Brookline I stopped in at Eureka Puzzles and found a Down Under obstructed-entry tray packing puzzle by Siebenstein Spiele. Down Under also poses some anti-slide type challenges. |

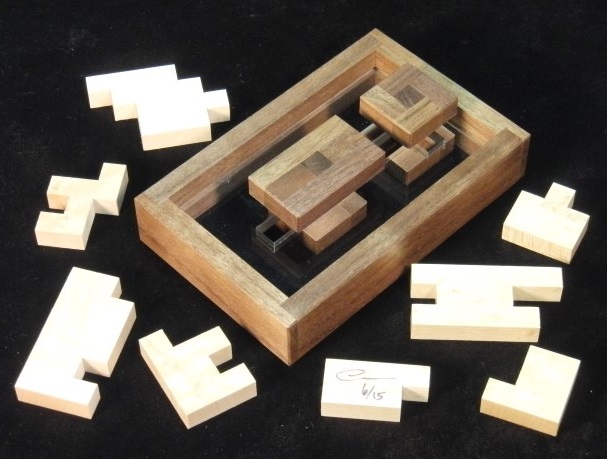
A-Pack - designed by Terry Smart and made by Eric Fuller from Walnut and acrylic with Bird's Eye Maple pieces. A packing puzzle with two trapped sliding shuttles. |
||



Core - designed by Yavuz Demirhan, made by Eric Fuller from Sapele and Cocobolo. Fit five pieces into a 3x3x3 cavity in the frame, working through a 2x2x2 opening. |
|||



Boussole - designed by Yavuz Demirhan, made by Brian Menold from Poplar and Cocobolo Fit four pieces into the frame. |
|||


Lockdown (271A) - designed and made by Stewart Coffin exchanged at IPP35 by Rob Jones Build a pyramid from the three pieces, within the tray - no force required. |

Checkbox - designed by Goh Pit Khiam and made by Brian Menold, from granadillo, ash, and east Indian rosewood. |
||


Tom's Square Dance, designed by Tom Jolly, made by Eric Fuller, from Padauk and Holly woods. This design is difficult to classify - the objective is to remove the nine pieces from the frame, then re-assemble the puzzle. The pieces interlock with each other and the frame via tabs and grooves. It seems like a sliding-piece puzzle but it really isn't, though its solution does depend on finding a sequence of correct movements of the pieces. |
 Open Window, designed by Tom Jolly, made and exchanged at IPP32 by Tim Udall Similar in principle to Square Dance, but only four pieces in the frame. |
||

Road Blocks - designed by Goh Pit Khiam made by and purchased from Tom Lensch. Fit the four pieces in the tray. Not easy, of course! Road Blocks received a Jury Honorable Mention in the 2015 Nob Yoshigahara Puzzle Design Competition |


Stumbling Blocks - designed by Goh Pit Khiam, made by Tom Lensch from Maple and Jatoba |
||

Rectangle Block (II B Flat) - designed by Christophe Lohe and made by Brian Menold. Sipo box with Olivewood/Makore pieces. Extract the four pieces from the frame, then reinsert. |
|||

Triagonal Agony designed by Laszlo Molnar, made by Brian Menold from Redheart and Yellowheart Pack six pieces in to the container to form a solid 3x3x3 cube inside. This must be done sequentially, through only two triangular openings. Shipped unsolved. I really enjoyed this one! |
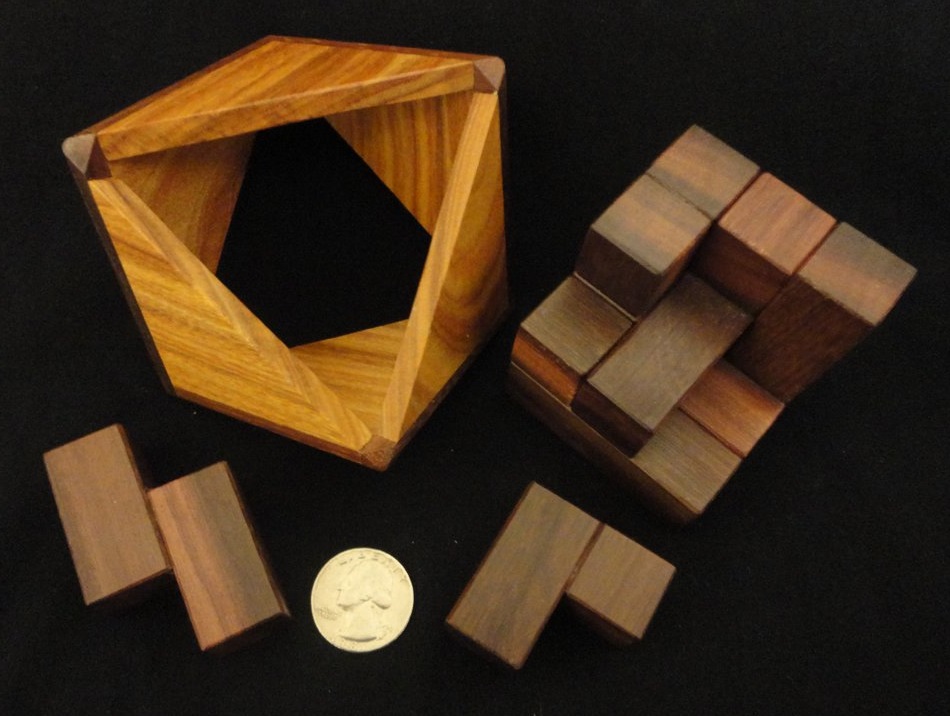
BDSM designed by Laszlo Molnar Made by Brian Menold Sequentially pack the 6 pieces into the cage through the openings. |
||

L-I-Vator I - designed by Laszlo Molnar, made by Brian Menold from Canarywood, Walnut, and Mahogany |

L-I-Vator II - designed by Laszlo Molnar, made by Brian Menold, from Redheart, Tree of Heaven, and Wenge. |

No Holes Barred - designed by Laszlo Molnar of Hungary, made by, and purchased from Brian Menold at Wood Wonders of New Jersey. Made from Koa and Iroko. |
|


Tau - by Dr. Volker Latussek, made by Pelikan Latussek quote on Pelikan's site: “My new packing puzzle has a small Greek letter tau on the top and your task is to close the box. I have to thank Laszlo Molnar for the design of the box that he first used in his beautiful puzzle NO HOLES BARRED. My goal in designing my new packing puzzle was to use as few equal pairs of pieces as possible. TAU gets by with only four pieces to close the box. I think the solution contains the most beautiful movement of all my puzzles so far. I hope that the solution will surprise you as much as it surprised me when I discovered the movement.” |

ACDC designed by Laszlo Molnar Made by Brian Menold Sequentially pack the 8 pieces into the box through the opening. Two differently patterned solutions. |
||


Grip - designed by Tim Alkema, made by Eric Fuller from Mahogany and Ash woods. Maneuver three pieces out of the box (then back in). Level 15.4. |
|||


Clamp designed by Tim Alkema Made by Eric Fuller from Sapele, Maple, and magnets |
|||


One Hole - designed by Bram Cohen made by Eric Fuller, from Ash and Shedua Five pieces must be maneuvered out of and in to a box with a single hole. Level 6.2.3.4.5 |
|||


Sherlock - designed by Taylor Pabst, Tyler Somer, produced by Marbles the Brain Store |
|||


Five Duo Cubes - designed by Brendan Perez, made by Eric Fuller from Sapele and Walnut |
|||


Quattro Cube - designed by Peter Gal, made by Eric Fuller from Walnut and Ash The five pieces contain 26 units, so they can form a 3x3x3 with one void. There is a unique solution for the void at each position. The box can be packed only with the void in one particular position. |
|||

Straight4ward - designed by Laszlo Molnar, made by Brian Menold from Spalted Sycamore and Redheart |
|||

Slide Packing - designed by Hajime Katsumoto, produced by Mineyuki Uyematsu This one took the Puzzlers Award in the 2016 Design Competition |
|||

Penta in a Box - designed by Hajime Katsumoto, produced by Mineyuki Uyematsu |
|||


Caramel Box - designed by Yasuhiro Hashimoto and MINE Uyematsu 2013-2014 Pack either the three lighter colored pieces or the three darker colored pieces into the box. A very nice puzzle with a unique 2x3x3 metal container having one unit baffle. This puzzle was among the top ten vote-getters in the 2014 IPP34 London Design Competition. |
|||

4L Basket - designed by Koichi Miura, purchased from MINE Pack the four identical pieces into the box through the restricted opening. This puzzle won the Puzzler's Award/Jury First Prize in the 2019 IPP39 Kanazawa Design Competition. |

5L Basket - designed by Koichi Miura, purchased from MINE Fit 5 L-tricubes into the box. |

5L Box - designed by Hajime Katsumoto, purchased from MINE (Mineyuki Uyematsu) Pack the five identical pieces into the box through the restricted opening, and close the lid completely. This puzzle won Jury First Prize in the 2018 IPP38 San Diego Design Competition. |
|

4L - designed by Yasuhiro Hashimoto, made by Eric Fuller from Ash and Padauk. Just fit the four L-shaped pieces into the box, through the opening in the acrylic lid. What could be simpler? This puzzle received a Jury Honorable Mention at the IPP35 Puzzle Design Competition (the IPP hosted in 2015 in Ottawa by Brett and me)! |

Hat Trick - designed by Laszlo Molnar and made by Brian Menold from Maple and Katalox. Fit the six identical L-shaped pieces into the box using the T-shaped slot. Hat Trick was among the top ten vote getters in the 2019 IPP Design Competition. The Katalox pieces are surprisingly dense! |

Turning Quarter Hole - designed by Hajime Katsumoto, produced by MINE |
|
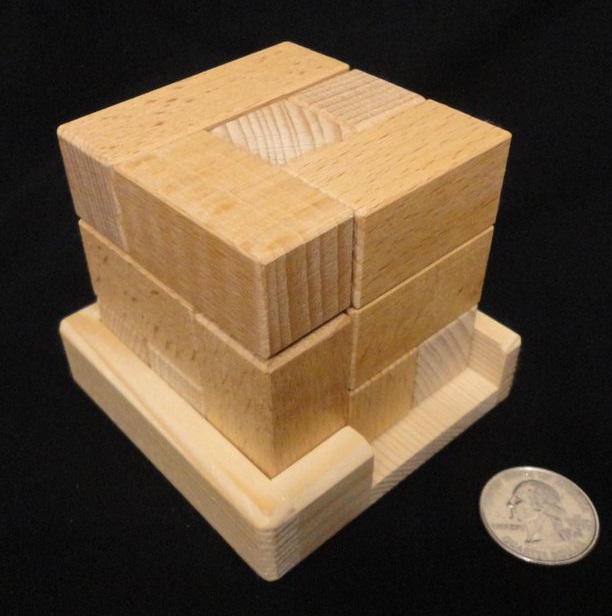



Stand By Cube No. 2 and Stand By Cube No. 3 - by Greg Benedetti In each cube, one piece is affixed to the base, and along with strategically placed openings in the base, constrain how you must build the cube. |
|||


Stand By Cube 4 - designed by Greg Benedetti, made by Eric Fuller from Leopardwood and Maple. 10 pieces assemble sequentially into the frame to form a 4x4x4 cube. |
|||


Loopy L Cube 1 - Pluredro |
|||


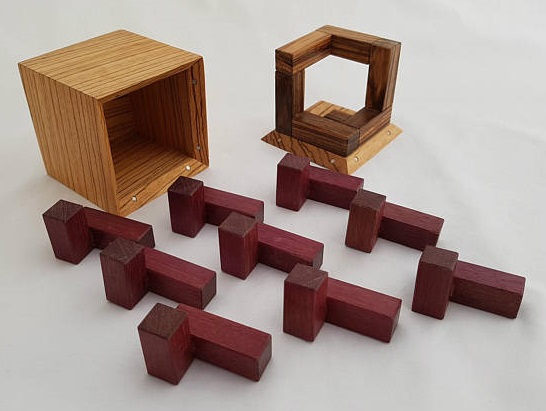
Looped Nine - designed and made by Yavuz Demirhan |
|||


N-Closed - designed by Haym Hirsh, made by Brian Menold from Marblewood and Wenge for the frame, and other exotic woods for the pieces.
Haym has this to say about the origins of this puzzle:
Haym's cage forces an interesting sequential assembly, and Brian has crafted a beautiful puzzle object. |
|||
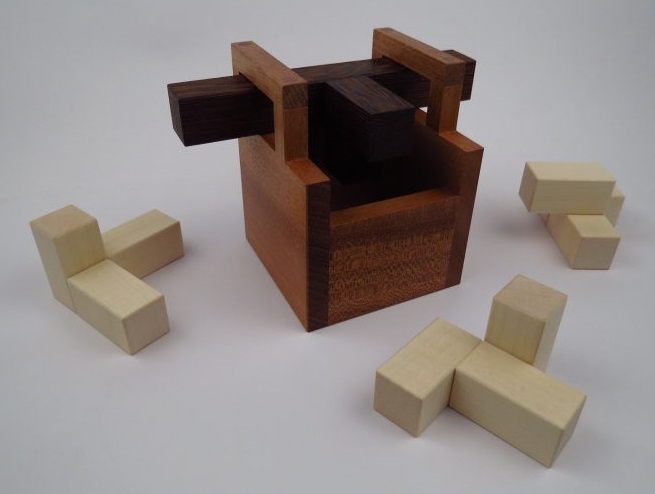
Wishing Well - designed by Alexander Magyarics and made by Pelikan from Mahogany, Wenge, and Maple. Fit the three pieces within the 3x3x3 cube under the 'timber' (the T-shaped captured moving obstacle) such that the timber ends up pushed back and horizontally centered as shown (it has a cubie that protrudes down into the 3x3x3 space). The 3x3x3 space need not be completely filled. Moves: 21 (7.6.8)

Pincers - designed by Alexander Magyarics and made by Pelikan from Cherry and Ovangkol. Fit the three pieces below the top layer. There is only one intended solution without rotations and the disassembly level is 9.4.3. I found a level 5 solution using rotations.
|
|||



Pepper Castor - designed by Alexander Magyarics and made by Pelikan from Zebrano and Padauk. Fit the three pieces into the container so all the windows are filled. So beautiful, even if I haven't solved it! |
|||

Alpacka #1 - designed by Alexander Magyarics and made by Brian Menold. Wenge box with Olivewood/Wenge pieces. Maneuver the six pieces U-side down into the box. |
|||

Roller Coaster - designed by Laszlo Molnar, made by Brian Menold from Brian's "Pajamawood" laminate. Put the pieces fully inside the box, without touching them inside the box. An entry in the IPP 2018 Design Competition. |

Casino - designed by Volker Latussek, made by Pelikan from Oak and Maple. Fit the six disks into the box, no forcing! This puzzle received the Puzzle of the Year, Jury Grand Prize, and Puzzler's Award at the IPP38 Puzzle Design Competition in 2018 in San Diego. |

Box With Two Balls - designed by Christoph Lohe, made by Eric Fuller from Ash, Zebrawood, Purpleheart, and Padauk. |
|


Euklid - designed by Volker Latussek Note that the photo of the packed puzzle is not a solution - the seven pieces must not stand proud of the box. |

Euklid for Kids - designed by Dr. Volker Latussek, made by Pelikan. Fit the three pieces into the restricted-opening box. How hard could it be? |
||


Pin Block Case - designed by Hajime Katsumoto, made by Eric Fuller from Maple and Walnut. Fit the four pieces into the box such that no pins or slots show. Very satisfying! |

X Cage - designed by Frederic Boucher, made by Eric Fuller from Walnut and Yellowheart. Fit five pieces into the cage via the opening in the acrylic top. |
||

Cubyful 2 - designed by Lucie Pauwels, made by Eric Fuller from Leopardwood, Wenge, and White Oak. Fit the seven loose pieces into the box, working around a movable but captive piece. |

Half Lid Box - designed by Hajime Katsumoto, made by Eric Fuller from Sepele, Yellowheart, and Bird's Eye Maple. Fit the five loose pieces into the box, working around a movable but captive half lid. |
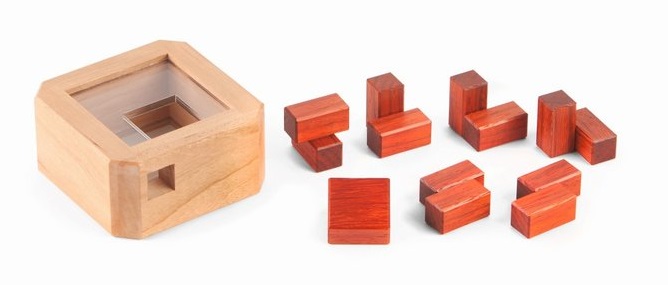
Marble Cake Plus - designed by Christoph Lohe, made by Eric Fuller from Cherry and Padauk. |
|

3-in-1 - designed by Goh Pit Khiam and made by Tom Lensch Three different sets, each of five identical pieces, can be fit separately into the tray. |
|||
|
Two designs from Goh Pit Khiam, made by Tom Lensch.
Both are restricted-opening sequential packing puzzles - Airlock and Bottleneck:
|
|||

Packing Puzzle 4P, Problems 1 & 2 - designed by Hajime Katsumoto 2017, purchased from MINE Pack the four identical flat acrylic P pentomino pieces into the box through the restricted openings, then flip the frame over and do the same on the other side.
Legal Packing - designed by Koichi Miura, purchased from MINE Exchanged at IPP39 by Ryuhei Uehara. Pack the six acrylic pieces into the tray through the restricted opening with no force. The pictured packing is illegal because it uses force.

Pocket - designed by Koichi Miura and Mineyuki Uyematsu, purchased from MINE Separately pack each set of four acrylic pieces into the tray through the restricted opening.

Tetra Spinner - designed by Yasuhiro Hashimoto and Mineyuki Uyematsu, purchased from MINE Pack the five acrylic tetromino pieces into the frame through the restricted opening. The white frame is free to move about between the clear plates trapped only by the central pillar.
|
|||
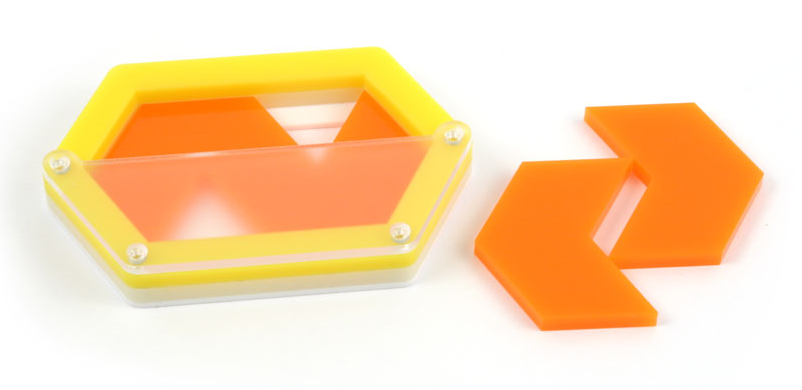
Croissant - designed by Koichi Miura, produced by MINE Among the Top Ten Vote-Getters in the 2021 IPP Puzzle Design Competition. |
|||

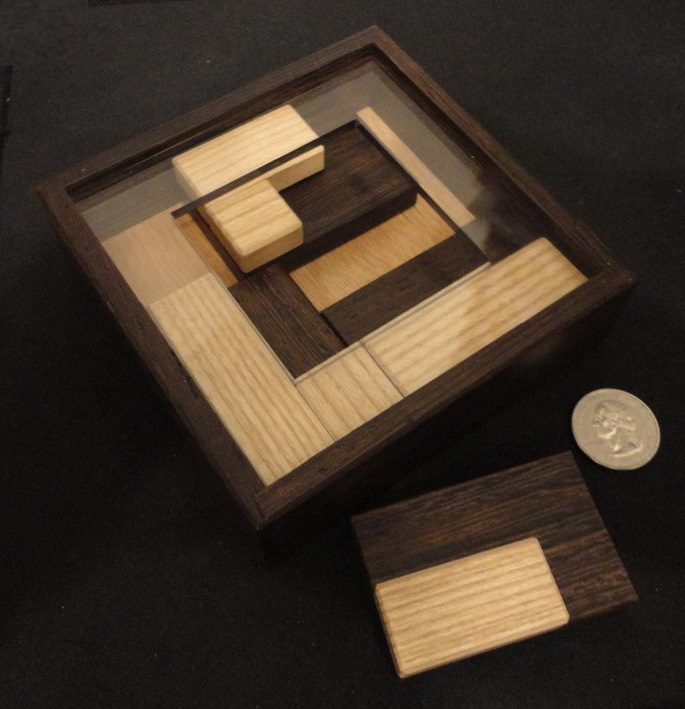
Squary Pack 6 (2x2 sized tiles) and Squary Pack 3 (2x3 sized tiles) - by Yavuz Demirhan Sequentially pack/slide the four 2-layer pieces into the tray through the restricted opening. A nice novel mechanism by Yavuz. Check Yavuz' Etsy shop Cubozone. |
|||

Quadro - by Yavuz Demirhan at CubozoneTR |

Four Cubes - by Yavuz Demirhan at CubozoneTR |
||


Arko - by Yavuz Demirhan at CubozoneTR |
|||
Corner Cube - by Andrew Crowell Sequentially pack the pieces into the box through the restricted opening (which is a weird size) such that the open corner will be filled. Of course rotations will be required! Check Andrew's Etsy shop arcWoodPuzzles. |
Edge Cube - designed by, made by, and purchased from Andrew Crowell in his "ARCparent Cube Series" A restricted-opening sequential packing puzzle, similar to Osanori Yamamoto's designs - one must fill the opening - but Crowell of course requires rotations! |

Angle Cube - from Andrew Crowell |
|
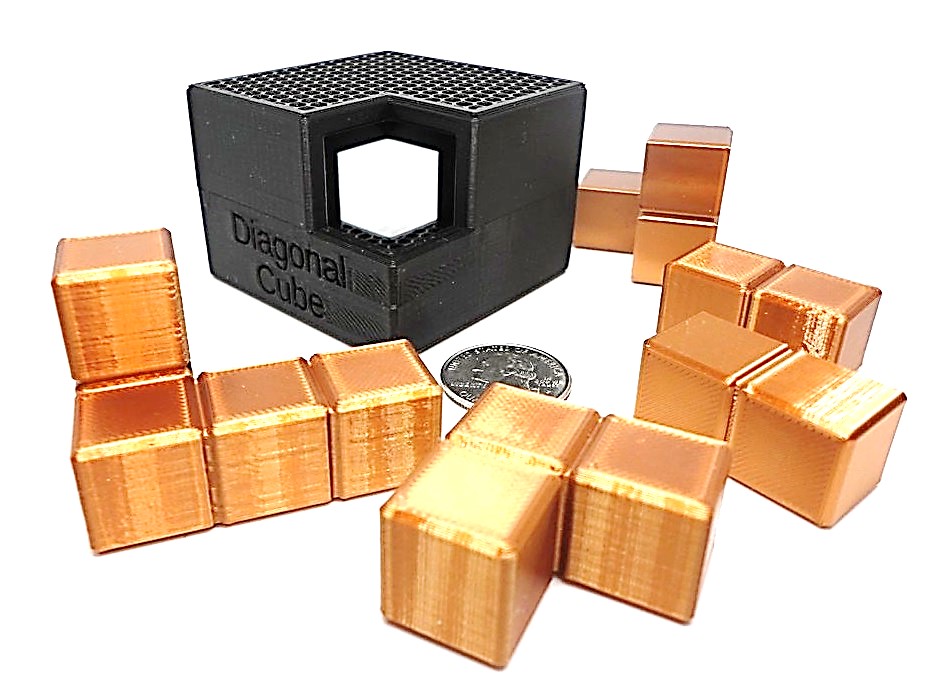
Diagonal Cube - designed by Andrew Crowell Pack the four pieces into the box which has two diametrically opposed restrictive openings. No gaps should be visible. Thanks, Brett! |

Acorn 1 & 2 - from Andrew Crowell |
||

Turn Them In - pack four pieces in the box and screw closed the lid, designed by Theo Geerinck and Symen Hovinga I purchased a 3D printed instance using Treatstock |

Hive Mind - by Alan Lunsford at layerbylayerpuzzles on Etsy. |

Soma Licious - designed and 3D-printed by Ken Irvine The Soma Licious is a new way of forcing a sequential packing of the Soma pieces with dimples into a box with internal spikes. |
|
|
I bought a subset of the Picnic Basket puzzles 3D printed from the Etsy shop of
Akaki Kuumeri
I chose three-piece puzzles including Cake (pink, easy), Hamburger (brown, medium), and Subway (gray, hard). The baskets are optional so you can get just one. I really like these! Tricky but tractable, each one requires some thought and planning and might not at first seem solvable. Brett printed me a nice set of Akaki's Picnic Basket puzzles:
|
|||

Opposite 1 & 2 - designed by Alexander Magyarics, made by Brian Menold Two sets of three pieces, each set in a different wood type. Use each set individually to pack the box such that all its external openings are filled. Each puzzle has a unique solution and in both cases getting the last piece in takes nine moves! |
|||


Kei Cube 1-1c, 1-4c, and 2-4c - designed by Hidekuni Tamura, produced by Puzzlewood Thanks, Chelsea! |
|||
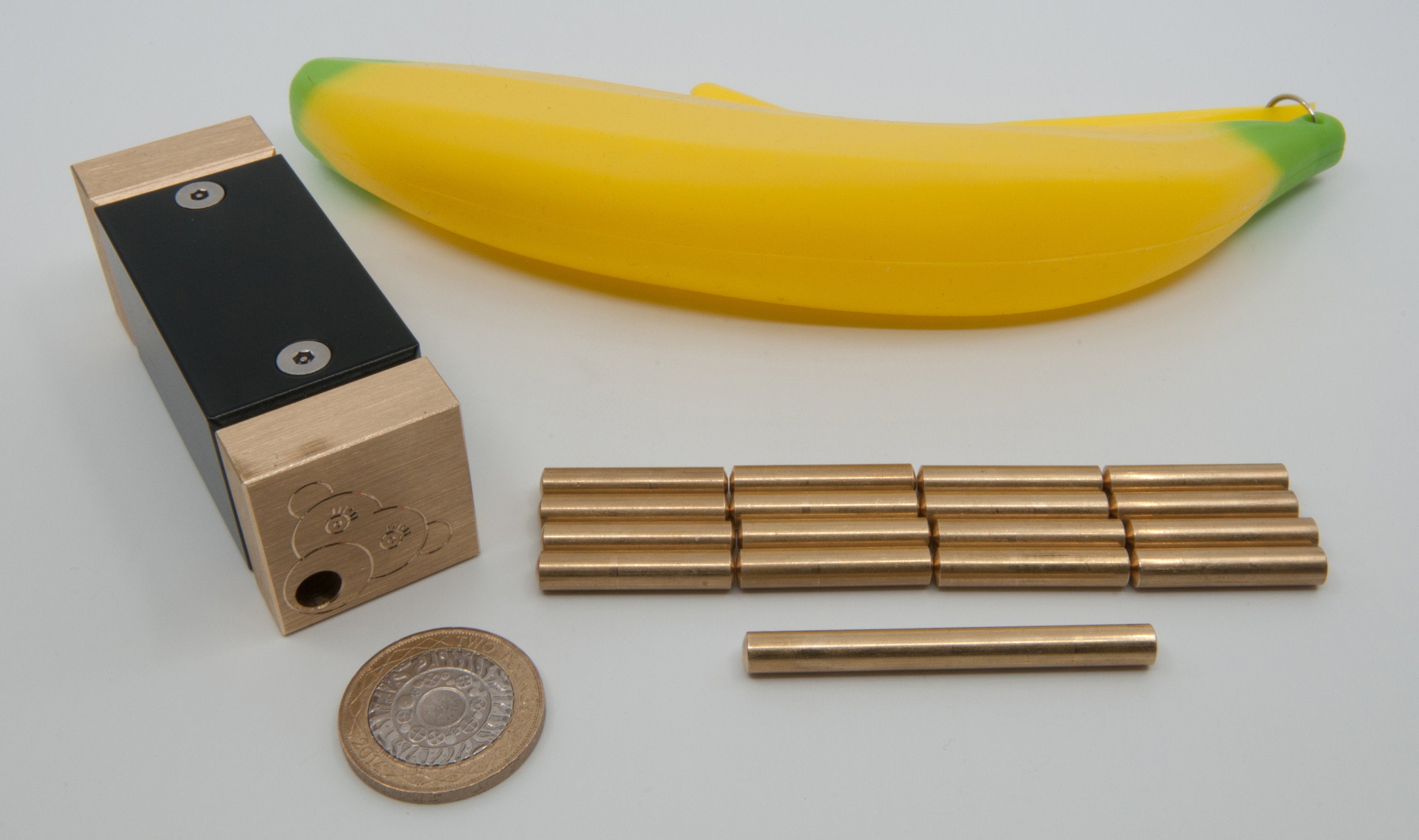
Feed the Monkey - designed and made by TwoBrassMonkeys Get the monkey to swallow all the bananas including the last long one. |

Tetrahedrane - designed by Oskar van Deventer, Produced by Two Brass Monkeys. Based on Oskar's "Screwballs" puzzle, which he describes on his Shapeways page as "an easy assembly puzzle, first designed and prototyped in May 2009. The puzzle has six identical rods with right and left-handed threads at the end, and four different [vertices], each with three threaded holes. The object of the puzzle is to assemble a tetrahedron. Because of the threading, the puzzle is assembled as an implosing, i.e. turning all the rods simultaneously and puzzling the balls together." |
||


TUTU - designed by Dr. Volker Latussek, purchased at Pelikan (out of stock) Pack the four pieces into the box through a T-shaped opening. I really enjoyed this one - I quickly formed a mental hypothesis as to how the pieces might go in, and it worked, but then I struggled a bit to learn the trick for extracting them. Now I can do it pretty smoothly - but lately the low winter humidity here (about 21%) is causing a U piece to bind. |
|||
This section is for Sequential Assembly and/or Restricted Opening puzzles designed by Osanori Yamamoto. He is one of my favorite designers!

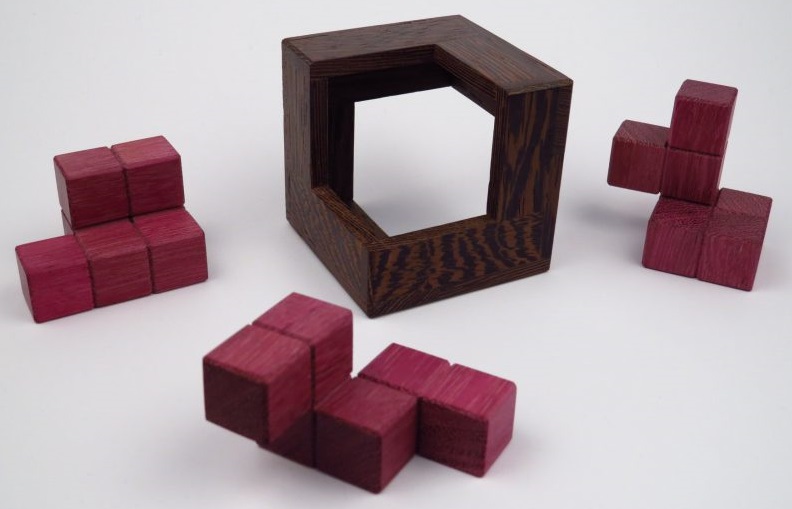
Belt Cube 3 (I got the version with a Purpleheart cage) - Yamamoto |


W Windows - Yamamoto |
|


Tulip 1 - Yamamoto |

Pack012 - designed by Osanori Yamamoto, made by Tom Lensch. Yamamoto-san always seems to come up with designs I like and this one does not disappoint! A restricted-opening sequential-packing using only three pieces. This puzzle was among the top 10 vote-getters in the IPP38 Design Competition. Picture taken on vacation in May 2019 from a deck overlooking the Atlantic. |
|

A trio of puzzles made by Tom Lensch, from Walnut and Yellowheart: (L to R) Petit Ring by Osanori Yamamoto - pack three pieces into the cage so no gaps show; Box Rebellion by Stewart Coffin - pack four L-tricubes in the box which is gated by a slightly-movable baffle; and Pack 3 by Osanori Yamamoto - pack 3 pieces into the cage so no gaps show. Sent unsolved - I managed to solve them all. |
||


Triple 4 - designed by Osanori Yamamoto and made by Yvon Pelletier, from teak and padauk. Not-a-solution shown :-) |

Galette - designed by Osanori Yamamoto Made by Tom Lensch Fit the five pieces into the case using the available openings. Galette was among the top 10 vote-getters in the IPP37 Design Competition. |
|



All Tetra Pod - designed by Osanori Yamamoto, purchased at Pelikan Made from Wenge and Padauk. Fit the eight pieces into the box. |
||
Many assembly puzzles use pieces themselves constructed from regular units - cubes, spheres, or tetrahedrons. An assembly puzzle can also have irregular dissimilar pieces. This section covers a wide variety of unusual assembly puzzle designs.



 Each Paracelsus Puzzle is one of a series of unique castings.
I have three - a Disk, an Oil Drop (or Waterfall), and Birds.
Each Paracelsus Puzzle is one of a series of unique castings.
I have three - a Disk, an Oil Drop (or Waterfall), and Birds.
These were made by Steve Johnson of Port Townsend, WA. The material is silicon bronze. I obtained a scan of a catalogue page showing seven designs:  I received a request for help in assembling the Waterfall puzzle.
Even though every Paracelsus Puzzle instance is unique, the following step-by-step assembly images of my copy might help:
I received a request for help in assembling the Waterfall puzzle.
Even though every Paracelsus Puzzle instance is unique, the following step-by-step assembly images of my copy might help:








|
|||||||||||
 Penthouse from Pentangle |
 Screwball (an oldie) U.S. Patent 3813099 - Scott 1974 |
 The "Moron Puzzle" To quote from the label: Morons - Take 1 Min. Idiots - Take 2 Min. Goofs - Take 3 Min. Numbskulls - Take 4 Min. |
|||||||||
 Bamboozle - B&P |
 5th Chair - Thinkfun (Gift from Brett) |
 The Chaotic Cube |
|||||||||
 2 Scheibenpuzzle (Logika) |

Heart - Logika |

4-piece puzzle - Logika |
|||||||||
 Think Tac Toe - Pressman |
 Pegged - B&P |
Olistripe The pieces interlock somewhat, but not enough. See U.S. Design Patent D500347 awarded to Daniel R. Oakley in Dec. 2004. |
|||||||||
 Think|a|ma|jig Copyright 1974 by Leonard J. Gordon (Gordon Bros.) |
 Jumpin' Frog Jumble The pieces do "interlace" but they don't really interlock in a solid 3D structure. |
||||||||||

 The Woody Cube (Nankai) - B&P |
 The Intragon from Naef Designed by Jost Hanny in 1989. Twelve pieces assemble inside a frame. See the Intragon pieces here. |

Six Key Mine (B & P) An R.D. Rose design. First Prize, 2003 IPP Puzzle Design Competition. The pegs have tongues that can interfere inside the sphere. Insert all 6 without interference. |
|||||||||

Just Fit - William Strijbos 16 pieces plus tray. Create a two-layer 5x5 checkerboard in the tray. 1990 Hikimi Wooden Puzzle Competition winner. |

Diamond Mind - Constantin |

Diamond Soul |
|||||||||
 Hippo Haven (Thinkfun) Each Hippo has two pegs. The pegs and holes in the base are of 4 different depths. Find a way to fit the Hippos in the base so all pegs are completely inserted. |

Short Circuit Purchased the Constantin version at GPP. Similar to Hippo Haven. |

Hooked Cube Philos (Goetz) |
|||||||||


Juha Six J's Cube set - IPP19 Together, the 24 pieces from the four cubes can make over 200 assemblies. |

Ziggurat - Creative Crafthouse has it. |
||||||||||

Tower of Babble by Leonard J. Gordon, Fair Oaks CA. - Item No. 134 I found an example in its original package. No date on the package. |

Thank You to Gus, who sent me a copy of his 3D-printed and improved re-creation of the vintage Tower of Babble puzzle. (Gus's pieces are closed and so don't clue orientation as much as the original design which has open bottoms). Tower of Babble is mentioned in Kevin's Puzzlemad blog post Top Ten(ish) of 2021. |

The Infernal Triangle was issued by Gordon Bros. and is marked "Item No. 135 1974 Leonard J. Gordon." The seven pieces are similar to those of the Tower of Babble, but here you must arrange them to form a two-layer triangular grid with 5 cylinders along a side. |
|||||||||
|
For anyone who may need help re-assembling the Tower of Babble (I am looking at you, M.D.) here is an assembly series...
|
|||||||||||

Harry Potter Mirror (see U.S. Patent 6976678 - Setteducati 2005) |


Punch Cards Tom Lensch |

A set of McDonalds promotional puzzles |
|||||||||

Link Puzzle make a cube from the loop of chain links |

Rising Mountain |
 This is a sculpture made of South Australian Red Gum wood by Robin Turner. I believe it is one of his "Ayers Rock" series. |
|||||||||

Impuzzleble |



A set of vintage puzzles from Plas-Trix of Brooklyn NY, includes: Krazee World, a pair of Batee Baseball, a pair of Krazee Links, a checkerboard dissection, a dissected scene |

Nuts and Bolts - Learningsmith |
|||||||||

Tool Trouble 1996 Great American Puzzle Factory, Norwalk CT. Assemble the 17 irregular pieces into a 7" x 9" (4x4 piece) rectangle. Six of the pieces have diagonal edges. Each piece depicts some tools, but they have nothing to do with the solution. |

Prismentwist - Logika |


Tuned In Milton Bradley 1973 Using all 14 gears, assemble a gear-train linking the knob with the male and female symbols. |
|||||||||

Chess Cubes |

Daily Mail Crown Puzzle |
||||||||||
| There have been several puzzles produced based on the theme of two sets of copies of distinctly-shaped pieces, where one set can be used to completely cover the other set (i.e. the sets cover the same area). Some of these are very challenging! This "Cover-Up" category seems to have been invented in 2004 by Robert Reid. | |||||||||||

Cover Up (2004) Puzzle-friend Jacques Haubrich kindly sent me a copy of this 8-piece puzzle, which he says is the "mother" of this type of puzzle, designed by Robert Reid. |


Cover It Up Designed by Robert Reid; this was Saul Bobroff's exchange puzzle at IPP26, where it won an Honorable Mention in the Design Competition. Cover the dark pieces completely with the light pieces, no overlapping the darks. The total area of dark and light each equals 4x7=28 units. It should be possible... |

Boston Cover Up - designed by Robert Wainwright |
|||||||||

Top This! - Thinkfun This Thinkfun puzzle offers a set of challenges similar to Cover It Up and Boston Cover Up, but simpler. |

A Cover-Up variant by Krasnoukhov Purchased at IPP 29 in SF |
||||||||||

Erich Friedman's Cover Up design - three challenges. From Creative Crafthouse |

Another Cover-Up variant by Krasnoukhov I don't have this - photo from Lilly Library Slocum collection. I assume there are 3 of each piece. |
||||||||||
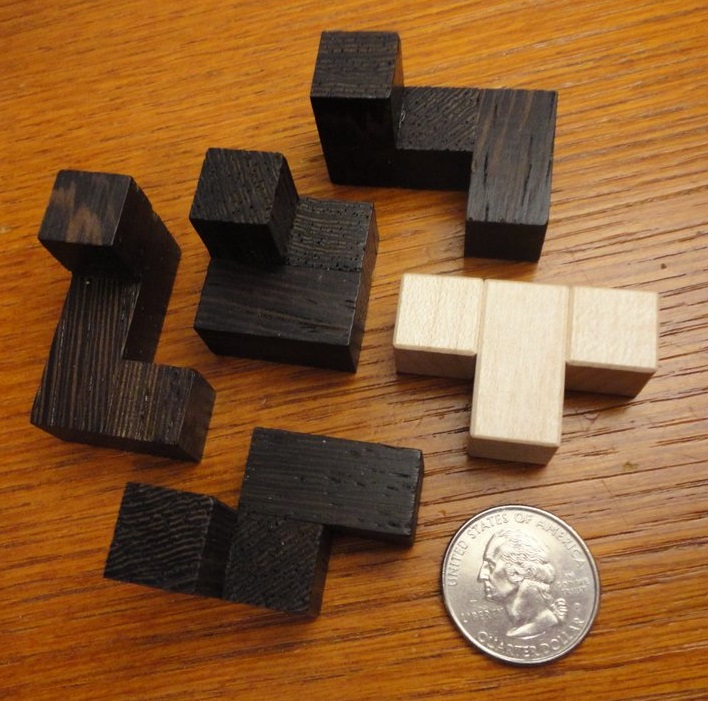
Cover Up - designed by George Sicherman, made by Brian Menold at Wood Wonders. Put the light piece on the table and cover it with the dark pieces (the table surface counts as covering). An entry in the IPP39 Design Competition. |
|||||||||||


MetallWürfel - Constantin |

Surface |

Times Square - B & P |
|||||||||

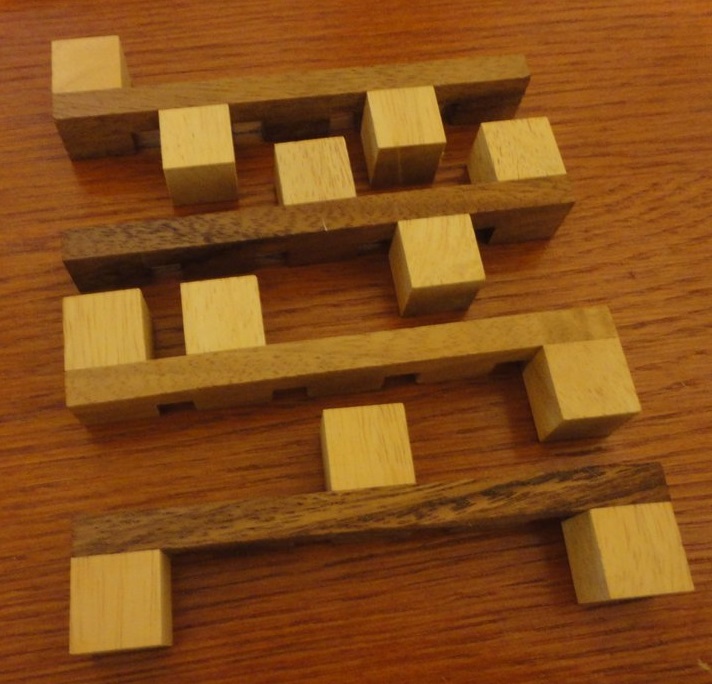

Surface (aka Parquet) An eight - piece weave type puzzle - each piece has three cubes affixed to a notched plank (all planks are the same). I received this in a group of puzzles with no provenance - it seems like the Bits and Pieces product "Times Square" but I have seen this puzzle offered from various places, including a Constantin version. I have another one with metal planks and colored cubes. |
|||||||||||


Dizzy Tower - Dizzy Art 1996 |


Naef's Discon puzzle, designed by Jost Hanny. Also, Discon Fever - a copy of Discon from B & P Peter Kaldeway's site shows a solution. |

I received this nicely made copy of the Discon puzzle, from craftsman Steve Kelsey. Thanks, Steve! |
|||||||||

Barricade - B & P |

This is Mental Block Puzzle #5 Vortex, by R. D. Rose. It is crafted from aluminum and comprises five rings with various pegs and holes around their perimeters, which must be assembled into a cylinder. |

MT5T (Make the Five Tetrominoes) - Mission 1 designed by MINE (Mineyuki Uyematsu) Arrange the four large pieces so that a subset of their gaps exactly enclose the five tetromino pieces. A similar version won a Jury First Prize at the 2011 Nob Yoshigahara Puzzle Design Competition |
|||||||||
 Idea Cube - by Idea Ocean |
 A paper version of Deep Sea Tango - obtained from George Hart at the 2007 NYPP. |

The 3Q Cube designed by Takeyuki Endo. Fit the three two-cube pieces into the cage. 2 solutions. |
|||||||||
 Milton Bradley made a couple of "Stickler" puzzles. Insert pins into a stack of disks which have holes at various points. The disks must be aligned so that all pins can be inserted. |
 Schalenwurfel - Logika |

Keiichiro Ishino modified Takeyuki Endo's 3Q Cube so that it has only one solution. A gift from Bernhard Schwietzer, at NYPP 2008. Thanks, Bernhard! |
|||||||||


A selection of "Dicebox Mindbender" puzzles by Mi-Toys - Half-Cubes, Rod by Rod, and Stacked Sticks, purchased at Eureka, and Cube Mates, from Brett. Imported from China by CHH Games. |
 Three diminutive but colorful plastic puzzles from Germany - build a cube from six panels, build a cube from nine concave tricubes, and build a step pyramid. |

9-Post Packing Puzzle De Vreugd B & P |
|||||||||

IQ Cube - Brainbenders Eight cubes with tabs and slots. Make a 2x2x2. |

This is a relatively inexpensive mass-produced copy of Wayne Daniel's famous All Five assembly. Purchased from Mr. Puzzle Australia. |
||||||||||

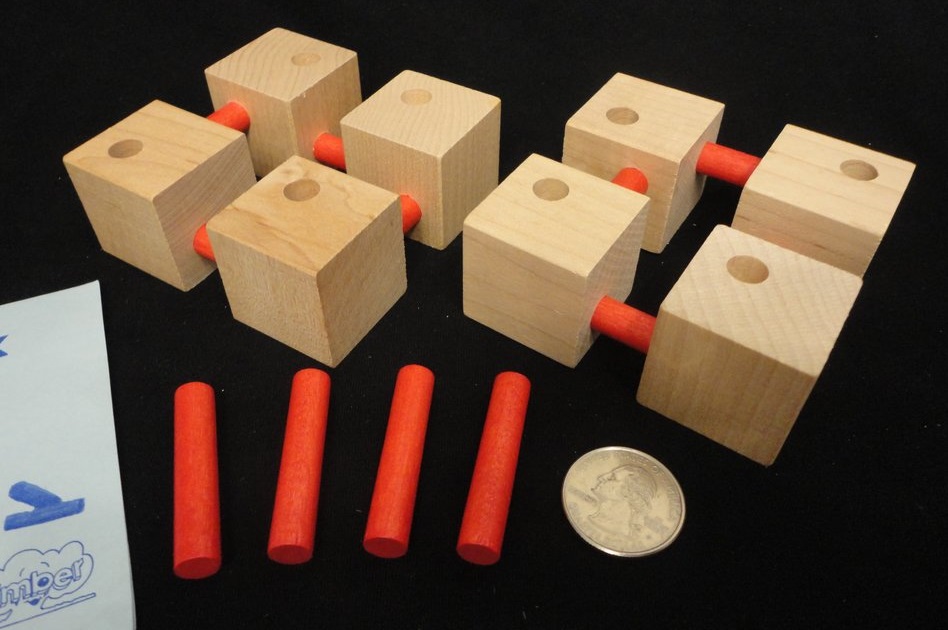
Mind Block - by t.c. timber, crafted by Habermaass Corp. of Skaneateles NY Eight cubes, each with three holes. Use twelve dowels to connect the cubes and build a 2x2x2 structure. |
|||||||||||


Dodec Puzzle - designed by, made by, and purchased from Simon on Etsy at SimonDSolidGeometry. A 3-dimensional edge-matching puzzle in the form of a hollow dodecahedron. Somewhat difficult to disassemble but comes with two wedges to help. |
|||||||||||
Here is a series of assembly puzzles by Andy Snowie:






 From left to right, they are: Orbsticle, ConeFusion, CyliPlex, EllipToy, and Pocket CalmPlex.
From left to right, they are: Orbsticle, ConeFusion, CyliPlex, EllipToy, and Pocket CalmPlex.
|
|||||||||||
 Jamaika - by Markus Goetz |
 Tirol Chocolate Purchased at IPP28 in Prague, from Wil Strijbos. |
 Octix - Trigam |
|||||||||
 Pairs of Prisms Ergatoudis IPP13 exchange |
 Trevor Wood's Prism Cube - unknown craftsman Made from highly figured canarywood. |
 3 Pyramid Cube by Philos |
|||||||||

 The Jeu du Cube and L'Enervant puzzles are vintage French non-cartesian cube dissections. (I believe Le Tracassier is also the same set of six pieces.) |
|||||||||||
 Obsivac Cube 1 |
 Obsivac Cube 3 |


Naef Kniff by Manfred Zipfel and Cordula von Tettau (See Ishino's Kniff page.) Purchased at IPP28 in Prague. |
|||||||||
|
|||||||||||

 The Triangle Cube aka Pantene |
 The 3456 Pythagoras Puzzle from Pentangle challenges you to use the nine pieces to form a set of three cubes 3x3x3, 4x4x4, and 5x5x5, then add them together and form one 6x6x6. |
 Hexahedroom This very nice puzzle was made by Eric Fuller, from Ebony and Jatoba woods. Form a cube within the box by fitting the pieces in via the available holes. A cool solution. Based on an IPP25 exchange from Hirokazu Iwasawa. |
|||||||||

Olymp by JCC |

The Double Octagon Box from Bits & Pieces Same idea as the cereal box puzzles from Synergistics. |

peg square (not sure of name or manufacturer; it's not the Naef design) |
|||||||||

The Sticky Cube Designed by Bernhard Schweitzer A gift from Bernhard at IPP 29 in SF - thanks! |

This six-piece puzzle is a 3-D printed adaptation by George Bell, of Stewart Coffin's Peanut design (see the original in wood at PuzzleWorld, and at Scott T. Peterson's site). I ordered the 3 cm. version from George's Shapeways store, in Alumide material. (Photo by John Devost.)
Overall,
According to George Bell, the six pieces (or subsets of them) can form only a limited number of symmetric shapes
(but he doesn't know how many of each could actually be assembled,
since in some the pieces interfere with each other and won't slide together):
(1) 3-ball: 2-1 flat triangle;
(4) 4-ball: 2x2 flat square; diamond; "canoe" (almost corners of a tetrahedron) (5) 5-ball: 4-1 square pyramid; "bridge" (2-3 flat trapezoid); 2x2 square w/ one outlier; (19) 6-ball: 6-ball flat ring; 3-2-1 flat triangle; "arch" (2x3 chevron - NOTE: the 2x3 rectangle is impossible); "boat" (flat 2x2 square w/ two opposite outliers); "cannon" (4-1 square pyramid w/ one outlier); octahedron (2x 2-1 flat triangles, packed vertically); "squashed octahedron" (2x 2-1 flat triangles in two offset layers); There are many more asymmetric shapes. |
||||||||||

Only 2 Sticks designed by Kofuh Satoh and made by Saul Bobroff Purchased at NYPP Feb. 2010 |
|||||||||||

Holzwurm (Product No. 6038), from Philos. Designed by Dieter Matthes. Form a 3x3x3 cube from 9 pieces having protrusions and hollows. Purchased at The Games People Play. |

Twist-L-Dan, in Oak, Wenge, and Karin woods, designed by Takeyuki Endo. Purchased from the Karakuri Club. |

8Pd, in Oak, Angsana, and Karin woods, designed by Takeyuki Endo. Purchased from the Karakuri Club. |
|||||||||

One Four All & All Four One Made by and purchased from Mr. Puzzle Australia. I traded this and no longer have a copy. Designed by Arcady Dyskin and Pantazis Houlis Entered in the IPP30 2010 Nob Yoshigahara Puzzle Design Competition, where it placed in the top ten. Arrange the four pieces (representing the three Musketeers plus d'Artagnan) in the frame so that they are self-supporting in the frame - you must be able to handle the frame without the pieces falling out. The frame is made from Queensland Blackbean with Queensland Silver Ash joins. The pieces are made from Queensland Silver Ash, Papua New Guinean Rosewood, Western Australian Jarrah & Queensland Blackbean. |

Yubisaki Annai - Takeyuki Endo - IPP30 Fit the six 1x1x2 blocks into the cage. Five blocks each have a protrusion that will interfere with other blocks and the cage. |
||||||||||
|
A set of three Star Wars themed Pizza Hut (South American) promotional puzzles from 1997 -
the Death Star (interlocking/assembly); Han Solo frozen in Carbonite (sliding piece); and R2D2 fixing C3PO (dexterity): 


|

Promotional puzzle from IBM What solid shape will fit through each hole, completely filling the outline? |
||||||||||
 Tri-Bal Trifle, designed and exchanged at IPP32 by Rob Hegge, made by Formulor Assemble the pieces so that the triangular armature balances. |


Knobeltorte A put-together puzzle in an egg. Layers of pieces with indents and knobs similar to the Prairie Dog Town puzzle. |
||||||||||
 Full Bloom, designed by Ferdinand Lammertink, made and exchanged at IPP32 by George Miller Eight rings, each having two petals in various positions. Stack the rings so that no petals overlap. |
 Jerrymander, designed and exchanged at IPP32 by Bill Cutler, made by Laser Perfect |
 Washington DC Sightseeing, designed by Tania Gillen, made and exchanged at IPP32 by Marcel Gillen |
|||||||||
 DC Tease, designed, made, and exchanged at IPP32 by James Kerley |
 Tantalizing, designed, made, and exchanged at IPP32 by Yee-Dian Lee |
 Shameful Congressional Gridlock, designed and made by Vaclav Obsivac, exchanged at IPP32 by Patrick Major |
|||||||||
|
|||||||||||
|
GeoBrix - at first sight seems to be a standard 2D tray packing puzzle.
There are thirteen substantial-sized pieces - each a black solid planar polycube with one colored face. They include some tetracubes, pentacubes, a hexacube, and a septacube. However, not only is there the expected challenge to fit the pieces back into the square 8x8 tray (for which there are at least 18 solutions) but an included booklet gives 20 different tangram-like silhouettes to be built from the pieces. And finally, one can build a 4x4x4 solid cube from the pieces. Lots of replay value here. Solutions are included. 

|
|||||||||||

By the Book - Brainwright A series of graduated challenges - stack specific books (and usually a cat) between two shelves such that the top shelf is level. Level (flower pot) included. |

Restricted Area - designed by Stewart Coffin, made and exchanged at IPP35 by Saul Bobroff. |

Double Feature - designed by Stewart Coffin, made by Saul Bobroff Pack in six pieces through the occluded aperture. Thanks, Saul! And very glad to see you up and about at IPP37! |
|||||||||

Michelin X - a vintage French advertising puzzle. Form an X from the pieces. Fit is imprecise. |

Michelin Tire - a vintage French advertising puzzle. Make a tire from the pieces. Fit is imprecise. |
||||||||||



Three Locked Cubes - designed by Tzy Hung Chein and made by Brian Menold from Bocote A great little three-piece puzzle to bring to get-togethers. Finally picked one up in this round. |
|||||||||||

3 Cubes - designed by Ichiro Kohno, made by Ken Irvine Arrange the 3 pieces to make 3 identically sized cubes simultaneously. Among the top 10 vote-getters in the IPP38 Design Competition. Gift from Ken at RPP2018 - Thanks! This seemingly simple puzzle had me stumped for *way* too long! Some folks get it readily, but not I! |
|||||||||||

The Colonel's Bouquet - designed by George Sicherman and made by Brian Menold from Honduras Rosewood Four hexacubes - combine any pair to make a symmetrical shape (each pair has a unique solution). Also, all four pieces make a 2x3x4 block. An entry in the 2017 Design Competition. |
|||||||||||


Gold Bar Puzzle - Japan Assemble the bar in a supplied flimsy flocked plastic tray, from 30 pieces. Each of 15 different pieces are duplicated, and the rounding of the bar at its corners and along its top edge, as well as its sloping sides, conspire to make this assembly much easier than having 30 pieces would suggest. I don't know how Indiana Jones may tie in to the puzzle, but his cross-eyed expression here is entertaining. |
|||||||||||

"Bokeless" - a quirky, weird set of press-fit plastic assembly puzzles from Japan. |
|||||||||||

Interlox - Brainwright Thanks, Alison! |

Arokah - Steve Brazier Use subsets of the (one-sided) tiles to cover a series of graduated challenge shapes. I found a review of Arokah on YouTube |

Podium - by Yavuz Demirhan |
|||||||||

Philos IQ Fit 3507 Rectangle (Rechteck) 10 pieces - all the ways two 1x2x2 blocks can be joined along at minimum a 1x1 overlap. This piece set has been employed in the Gemini puzzle designed by Toshiaki Betsumiya and issued by Naef. In Gemini the target shape is a 4x4x5 block. Stewart Coffin's Patio Block (STC #82) also uses these pieces, but omits the 2x2x2 and 1x2x4 and one other, substituting a duplicate of the "stairstep" piece to form a 4x4x4 cube. You can find a 3D print of Patio Block at Thingiverse.
Philos gives multiple objectives:
|
|||||||||||

Mouse Pack 3 - designed by Haym Hirsh Pack the three pieces into the sphere. A kind gift - Thanks, Haym! |
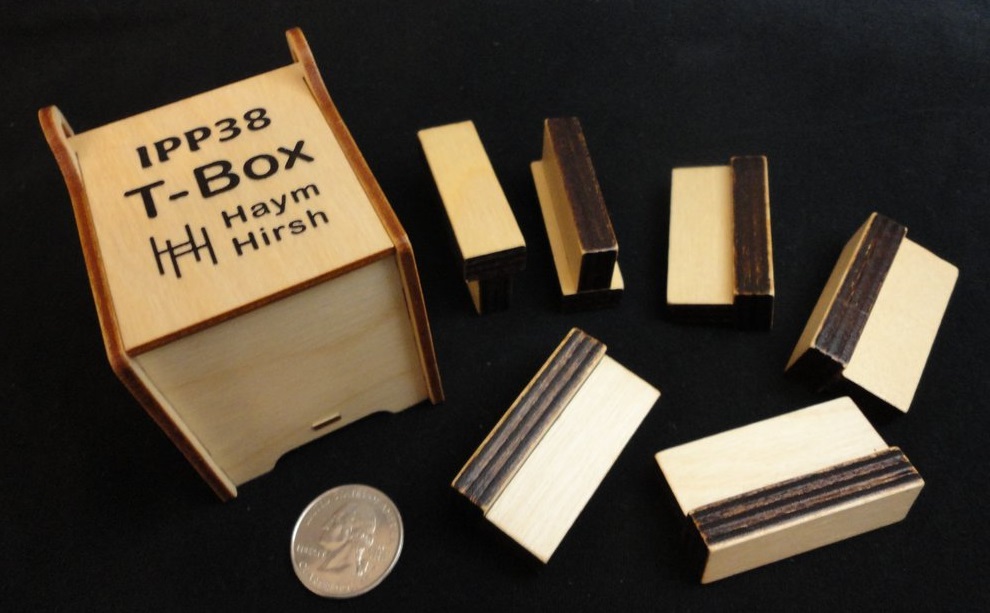
T-Box - by Haym Hirsh Pack the six identical T pieces into the box so the lid shuts properly. Thanks, Haym! |

Guillotine (aka Harun) - designed by Volker Latussek Six 'C' pieces and six 1x2x3 blocks can be packed into the box in two different ways so that the lid closes. Exchanged at IPP39 by Allard Walker A very kind gift - Thanks, Allard! |
|||||||||


Bubble - designed by Dr. Volker Latussek and made by Pelikan from Pear wood. Create two different free-standing structures from the four pieces such that the hemispherical divots form four internally hidden bubbles. Reminds me of Pentangle's Dragon's Eggs |
|||||||||||
Puzzle finger rings (Wikipedia article) made from several interlaced bands have been crafted by artisans from many cultures, and date back many years. The Puzzle Ring Store has a lot of info and a solution library.

This 4-band puzzle ring was included in the "De Luxe Puzzle Chest" No. 3006 from F.A.O. Schwartz. It's the Extraordinary Ring Puzzle No. 3522 by Shackman. Made in Japan. |

This 6-band puzzle ring was designed by Bram Cohen. It's 3-D printed. I bought it from Bram at IPP 29 in SF. |

Here is a 7-band puzzle ring I got in an auction lot. |
 Holistic Ring, designed and exchanged at IPP32 by Bram Cohen, made by Oskar van Deventer & Shapeways |
||


WOW5 (Wrap O-round Weave 5) - designed by Carl Hoff Made from cast silver by Jeff Bell ("puzzleringmaker") (Also available from Carl's Shapeways shop.) Unique amongst puzzle rings because in the solved configuration the five bands weave around each other all the way around the band rather than just along the top. WOW5 won a Jury Honorable Mention in the IPP36 Design Competition. WOW5 solution video on YouTube. |
||
An outfit called "Synergistics Research Corp." (New York, New York 10011), which evidently no longer exists, made several plastic assembly/packing puzzles years ago. I have not found an exhaustive list, but they include:
















Details of the Hershey's Kiss puzzle:





Here is an analysis I did of the Synergistics LifeSavers puzzle. I have found that all "flavors" use the same set of piece shapes. Each consists of 12 tori having combinations of pegs and holes. The tori stack together and fit into a cylindrical container approximately 55mm in diameter by 120mm high.
Each torus has a central hole immaterial to the solution. Each of the four cardinal positions (i.e north, south, east, and west) on its two faces may have one of the following features: This puzzle can be solved using PuzzleSolver 3D, if it is mapped to an analogue composed of unit cubes.
My mapping is straightforward but imperfect as it will allow "illegal" solutions -
fortunately the first solution produced is acceptable.
My mapping is as follows: rotate each torus depicted by 45 degrees clockwise. Use a 3x3 grid of cubes to model the torus
and any holes - delete a corner cube corresponding to any hole.
Leave the center cube filled in, to ensure the piece remains contiguous as required by PuzzleSolver.
Add a cube extending outwards for a peg, or two stacked for a double-length peg, at the appropriate corner positions on either side.
The target volume is 3x3x12.
The diagram shows the disks, and the mapping of each disk to cubes.
Two pieces are duplicated.
Solution number 1 is: 5, 2, 12, 9, 8, 6, 3, 1, 10, 11, 7, 4.
And here is an image of the solution #1, clipped from Puzzlesolver 3D:
This puzzle can be solved using PuzzleSolver 3D, if it is mapped to an analogue composed of unit cubes.
My mapping is straightforward but imperfect as it will allow "illegal" solutions -
fortunately the first solution produced is acceptable.
My mapping is as follows: rotate each torus depicted by 45 degrees clockwise. Use a 3x3 grid of cubes to model the torus
and any holes - delete a corner cube corresponding to any hole.
Leave the center cube filled in, to ensure the piece remains contiguous as required by PuzzleSolver.
Add a cube extending outwards for a peg, or two stacked for a double-length peg, at the appropriate corner positions on either side.
The target volume is 3x3x12.
The diagram shows the disks, and the mapping of each disk to cubes.
Two pieces are duplicated.
Solution number 1 is: 5, 2, 12, 9, 8, 6, 3, 1, 10, 11, 7, 4.
And here is an image of the solution #1, clipped from Puzzlesolver 3D:
 Synergistics isn't the only firm that made puzzles in the shape of food items.
Here are some additional examples...
Synergistics isn't the only firm that made puzzles in the shape of food items.
Here are some additional examples...
 I had a Parker Brothers' "Phony Baloney" when I was a kid - it disappeared but I found one in auction. |
 This miniature version of Phony Baloney was a cereal-box prize. |
 Here's one I found called the Banana Split, by Lakeside. |



Here is another Lakeside puzzle with a food theme - the Rotten Apple. Assemble the eight slices around the core so that the two "worms" can be inserted through the core to hold the puzzle together. The pieces have holes at 14 different heights, only two of which will line up with corresponding holes in the core. There are only two pairs of slices having core-aligned holes. Not difficult, but cute. |

 Prankfurter - Reiss |

 Burger Thing - Reiss |
 Here is a puzzle chocolate bar, the "Puzzle Bar" from Pentangle. |

 Another hamburger puzzle, made in China. |
 Here are Peter Piper's Fickle Pickles, a ten-piece packing puzzle. Made in Hong Kong, copyright 1973 Steven Mfg. Co. Discussed in Slocum and Botermans' The Book of Ingenious and Diabolical Puzzles on pp90-91. (Click the image to see the solution, cheater.) |
|